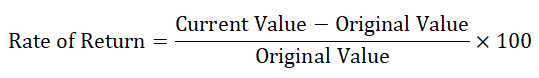Updated November 30, 2023
Definition of Rate of Return
When we invest money, we hope to make more money in return. That’s where the concept of “rate of return” comes in. To calculate the rate of return, we use the “rate of return formula.”
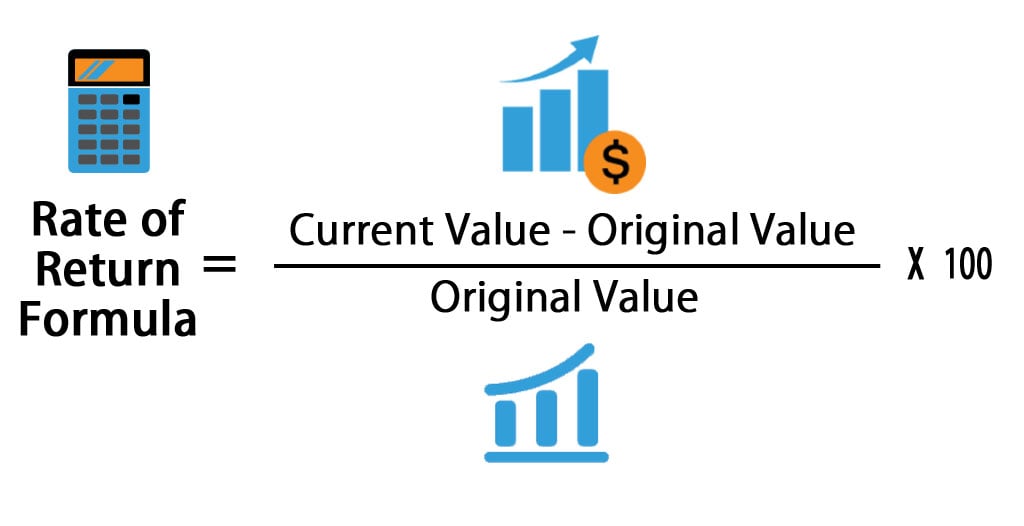
The rate of return formula tells how much money you made or lost on your investment over a specific time. The formula looks at how much money you initially invested and how much you ended up with and expresses it as a percentage.
If the return on investment is positive, you make a profit. But if the return on investment is negative, it means you lost money on your investment.
The rate of return formula is like a report card for investments. It helps investors know if they made or lost money on their investments. It helps them decide whether to keep investing or try something else to make more money.
Rate of Return Formula
Where,
- Current Value = Current value of the investment.
- Original Value = Value of investment.
The rate of return over one year on investment is known as annual return.
Importance of Understanding the Rate of Return
1. Helps evaluate investment performance: Corporations use the rate of return in finance in any form of investment like assets, projects, etc. By comparing the rate of return of different investments, investors can determine which investment is performing better and make informed investment decisions. Moreover, It is used in financial analysis by investors.
2. Enables risk assessment: It helps determine the risk factor in an investment. If the investment has a higher rate of return, it has a higher risk. But if an investment has a low rate of return, it has a lower risk. It helps investors decide how much risk they want to take with their investments.
3. Facilitates goal setting: The rate of return helps investors figure out how much money they need to reach their financial goals. By setting realistic goals and developing investment strategies, investors can use the rate of return to make sure they are on track to meet their financial goals.
4. Helps in portfolio management: The rate of return is important for portfolio managers. By looking at the rate of return for individual investments and the overall portfolio, they can make informed decisions about where to allocate assets and how to manage risk.
Types of Rate of Return
There are three types of Rates of Return that investors use to measure the performance of their investments.
#1 Simple Rate of Return
It measures the return on an investment in percentage terms, based on the difference between the current value and the initial investment, without considering the time factor. It helps evaluate the performance of investments with a short-term horizon.
#2 Compound Annual Growth Rate (CAGR) or Annualized Rate of Return
While the regular rate of return provides a snapshot of investment performance over a specific period, the annualized rate of return (CAGR) provides a more comprehensive view of how an investment has performed over the long term, factoring in the impact of compounding.
#3 Internal Rate of Return (IRR)
The Internal Rate of Return (IRR) measures and estimates the profitability of an investment or a project. It shows the discount rate at which the cash inflows’ net present value equals the cash outflows’ net present value or the rate at which the investment or project breaks even.
Here,
- NPV is known as the net present value of the investment or project.
- Cash Flows are the cash inflows and outflows for each investment time.
- We express IRR as a percentage.
- t is the period of the investment or project.
Rate of Return Formula in Excel (With Excel Template)
Example #1 Rate of Return on Investment
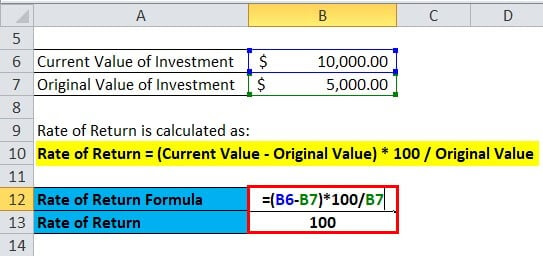
Steve invested $5,000 in 1,000 shares at $5 per share in 2021. He sold all his shares for $10 each in 2022. Find out the rate of return on the invested amount of $5,000.
Solution:
Implementing the formula, let’s find the ROR:
Putting the value in the above formula:
Rate of Return = ((10,000 – 5,000) / 5,000) x 100
= (5,000 / 5,000) x 100 = 100%
Thus, the rate of return on shares is 100%.
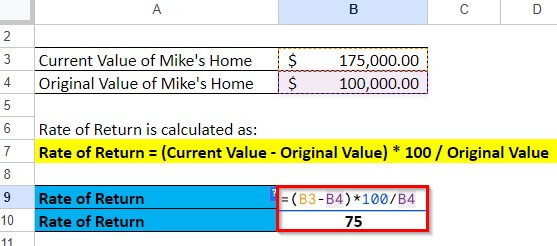
Example #2 Rate of Return on Property
Mike purchased a property on the outskirts of California for $100,000 in 2016. Due to rapid development in the region, the property’s value increased over the years. In 2022, Mike had to sell the property for $175,000 due to a job change. Let’s calculate the rate of return on Mike’s investment in the property.
Solution:
Let’s find his rate of return by implementing the formula:
Rate of Return = ((Current Value – Original Value)/ Original Value) x 100
Putting the values, we get the below result:
Rate of Return = ((175,000 – 100,000) / 100,000) x 100
= (75,000 / 100,000) x 100= 75%
So, after the evaluation, the ROR on Mike’s home is 75%.
Example #3 Annualized Rate of Return
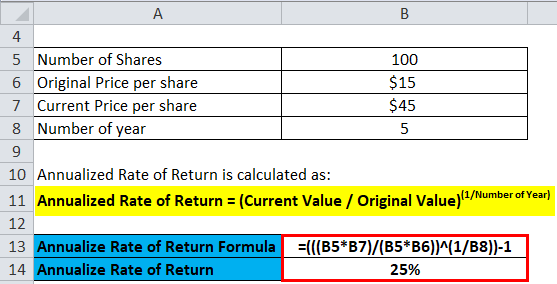
Smith purchased 100 shares for $15 per share and received a dividend of $2 per share yearly, and after five years, he sold them for $45. So, let’s calculate the annualized return for Smith.
Solution:
Let’s implement the formula and find the resultant,
Using the formula:
Annualized Rate of Return = (($45 x 100) / ($15 x 100))^(1 /5 ) – 1
= (($4500 / $1500) ^ 0.2) – 1 = 0.25
Thus, the annualized rate of return on shares is 25%.
Example #4 Comparing Rate of Return on Two Investments
Austin invested $1000 in shares of Apple Company in 2021 and sold his stock in 2022 at $1200. He then invested $2000 in the stocks of Google in 2021 and sold his stock in 2022 at $2800. Calculate the ROR.
Solution:
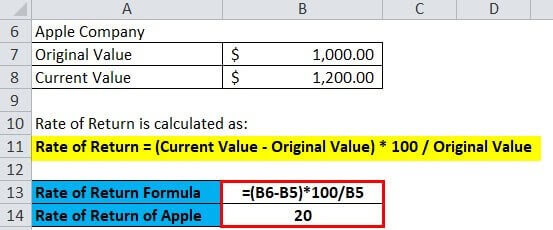
1. Calculating the ROR on the investment in Apple:
= ((1200 – 1000) / 1000) x 100= (200 / 1000) x 100
Rate of Return Apple = 20%
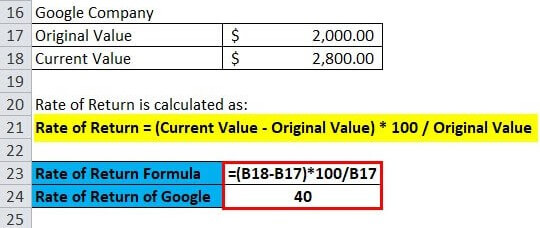
2. Calculating the ROR on investment in Google:
= ((2800 – 2000) / 2000) x 100 = (800 / 2000) x 100 = 40%
We can see that Austin earns more profit from Google’s investment than from Apple, as the ROR when investing in Google (40%) is higher than Apple’s (20%).
Example #5
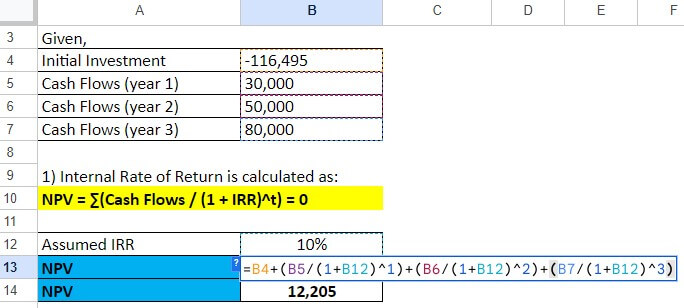
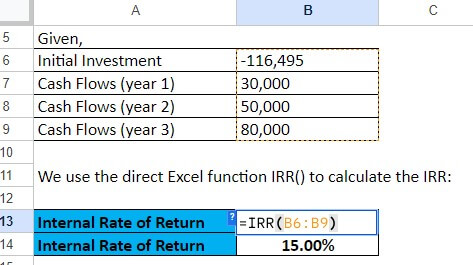
Steffi wants to invest in a project that will cost $116,495 upfront, and she expects to generate cash flows of $30,000 at the end of the first year, $50,000 at the end of the second year, and $80,000 at the end of the third year. Calculate the IRR.
Solution:
1. We will use a trial-and-error method to calculate the Internal Rate of Return (IRR). We set the Net Present Value (NPV) to zero using the formula:
NPV = ∑(Cash Flows / (1 + IRR)^t) = 0
By figuring out at what IRR value the NPV becomes zero, we can determine the IRR for this example.
Let us consider the first IRR as 10%:
NPV = -$116,495 + $30,000/(1+10)^1 + $50,000/(1+10)^2 + $80,000/(1+10)^3
= $12,205
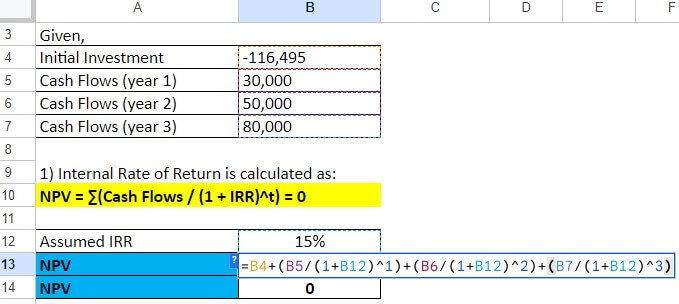
Let us try with IRR as 15%:
NPV = -$116,495 + $30,000/(1+15)^1 + $50,000/(1+15)^2 + $80,000/(1+15)^3
= 0
Therefore, the internal rate of return for the project is 15%.
2. We can also use the direct Excel function IRR() to calculate the IRR:
The Excel IRR() function takes the range of cash flows and the initial investment as the only function argument.
The formula will be: IRR(B6:B9)
Differences Between the Different Types of Rate of Return?
| Comparison | Simple Rate of Return | Annualized Rate of Return (ARR) | Compound Annual Growth Rate (CAGR) | Internal Rate of Return (IRR) |
| Definition | Considers total profit or loss | Measures the performance over a single year | Measures the average growth rate of an investment | Calculates discount rate where the net present value of cash flows is zero |
| Calculation | (Ending value – Beginning value) / Beginning value | ARR=(Ending value / Beginning value) – 1 | (Ending value / Beginning value) ^ (1 / Number of years) – 1 | Uses present value formulas to solve for discount rate |
| Factors | The time value of money is not considerable. | The time value of money is not considerable | It considers the compounding effect. | It takes into account the timing and amount of cash flows |
| Use | Used for short-term investments | Used for evaluating investment performance over a single year | CAGR is used for evaluating long-term investments | Used for evaluating investment opportunities and comparing project returns |
| Limitations | Ignores compounding effects, may not accurately reflect the overall performance | Ignores performance over multiple years | Assumes constant growth rate, may not reflect the actual performance | It may be difficult to calculate and may require specialized software or financial models |
Real-life Applications of the Different Types of Rate of Return
| Types of Rate of Return | Real-life Applications |
| Simple Rate of Return | Evaluate returns on individual investments such as stocks and bonds |
| Annualized Rate of Return | Evaluation of mutual funds, hedge funds, and other investment vehicles over multiple years |
| Compound Annual Growth Rate (CAGR) | Evaluating the growth rate of companies and investments over long periods and measuring the return on investment of a project or business venture |
| Internal Rate of Return (IRR) | Useful for capital budgeting for determining the profitability of investment projects |
Factors Affecting the Rate of Return
- Market conditions: The overall market conditions can significantly impact the rate of return of an investment. For example, most investments perform well during a bull market, while returns are generally lower during a bear market.
- Economic factors: Economic conditions, such as inflation, GDP growth, and interest rate growth, can also affect the rate of return on investment. Higher inflation or interest rates can reduce the value of an investment, while stronger GDP growth can lead to higher returns.
- Investment strategy: It can heavily affect the rate of return. For example, a conservative investment strategy that focuses on low-risk investments may generate lower returns than a more aggressive strategy that takes on higher levels of risk.
- Timing: Timing is another factor that influences the rate of return. For example, investing during a market downturn may lead to higher returns in the long run, while investing at the peak of a market cycle may result in lower returns.
- Fees and taxes: High fees or taxes can reduce the overall return generated by an investment. It can affect the overall performance of a company and even its future.
Example:
Ashley purchased a rental property for $200,000 in 2018 and sold it for $250,000 in 2022. Let‘s see how the above factors can affect her rate of return.
| Factor | Impact on Ashley’s Rate of Return |
| Market Conditions | If the real estate market was weak when Ashley sold the property, she might see lower returns than during a period of market upturns. |
| Economic factors | High inflation or interest rates during Ashley’s investment may reduce the overall value of her returns. |
| Investment strategy | If Ashley took on significant debt to finance the rental property, her returns might experience higher interest payments. |
| Timing | Ashley’s decision to sell shares in 2021 may have been influenced by timing, as the market was experiencing a strong recovery from the COVID-19 pandemic. |
| Fees and taxes | If Ashley paid high fees to her financial advisor or incurred significant capital gains taxes, it may reduce her overall returns. |
How Investors Can Use Rate of Return?
Investors can use the rate of return to evaluate the performance of mutual funds in which they have invested. Here’s an example of how an investor might use the rate of return to evaluate the performance of a mutual fund:
Example:
Suppose Max is an investor who has invested in a mutual fund with an initial investment of $10,000. After one year, the value of the mutual fund has increased to $11,500, including both capital appreciation and any dividends or distributions paid out during the year.
To calculate the rate of return for this investment, the investor would use the formula:
Implementing the formula, the ROR will be
Rate of return = ($11,500 – $10,000) / $10,000 = 0.15 or 15%.
So, the investor has earned a 15% rate of return on their investment in the mutual fund over the past year.
Max can compare the rate of return of his mutual fund to other benchmarks or mutual funds or, like the S&P 500 index or a similar investment index, to evaluate its performance relative to its peers. If the fund’s return consistently lags behind its peers or benchmark, Max may consider selling his shares and investing elsewhere or vice versa.
Rate of Return Calculator
| Current Value | |
| Original Value | |
| Rate of Return Formula = |
|
||||||||||
|
Recommended Articles
This has been a guide to a Rate of Return formula. Here we discuss its uses along with practical examples. We also provide you with a Rate of Return Calculator with a downloadable Excel template. You may also look at the following articles to learn more –