Updated July 29, 2023

Doubling Time Formula (Table of Contents)
What is Doubling Time Formula?
Doubling time, as its name suggests, is the time taken or the length of time your investment will double in size at some particular interest rate. This concept is also known as the Rule of 70 because doubling time can be approximate.
Calculated by dividing 70 by the interest rate. This will also lead to almost the same value as the doubling formula. This concept is common in comparing investments with different interest rates and helps us understand how quickly that investment grows.
Analysts and investors widely use this tool to evaluate investments like mutual fund returns, portfolio returns, etc. and can make appropriate decisions to achieve the target. For example, if you are an investor and upon doubling time calculation, you know your investment will double in almost 20 years. Now you can use this time to reduce, and your investment will be close to 15 years. You need to increase the rate of return on your investment. You can allocate changes to the portfolio to achieve that rate.
The formula for Doubling Time –
There are 2 ways by which we can find doubling time, and both will yield almost the same answer:
Where:
- Ln – Natural Log
- r – Interest Rate
In this formula, use the absolute value of r and not the decimal value. For example, if r is given 5%, we will use 5, not 0.05.
Examples of Doubling Time Formula (With Excel Template)
Let’s take an example to understand the calculation of the Doubling Time formula in a better manner.
Example #1
Find the time it will take to double your money if you can get a constant growth rate of 6%.
Solution:
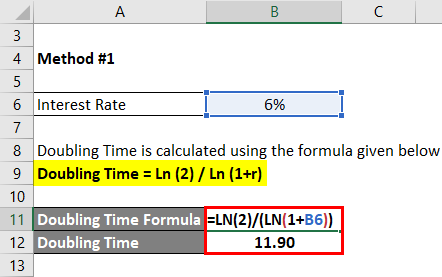
Doubling Time is calculated using the formula given below
Doubling Time = Ln (2) / Ln (1+r)
In this formula, use the absolute value of r and not the decimal value.
- Doubling Time = Ln (2) / Ln (1 + 6%)
- Doubling Time = 11.90 years
In Method 2, use the absolute value of r and not the decimal value.
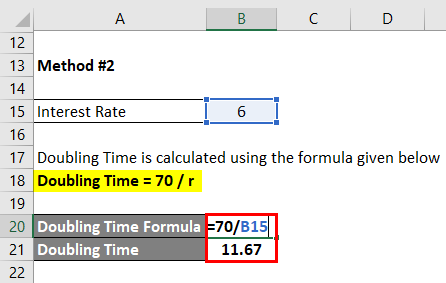
Doubling Time is calculated using the formula given below
Doubling Time = 70 / r
- Doubling Time = 70 / 6
- Doubling Time = 11.67 years
So if you see, both the formulas result in approx. Same answer, and if we round off the results, it will take us around 12 years to double the money given a 6% rate.
Example #2
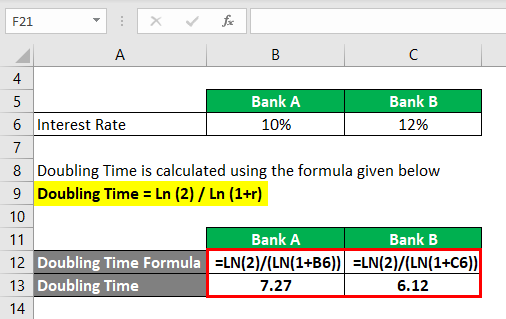
Let’s say bank A offers you a 10% constant interest rate if you invest your funds with them, and bank B offers a 12% constant growth rate. You want to see how fast your investment will grow and how long it will take to double your funds.
Solution:
Doubling Time = Ln (2) / Ln (1+r)
For Bank A:
- Doubling Time = Ln (2) / Ln (1 + 10%)
- Doubling Time = 7.27 years
For Bank B:
- Doubling Time = Ln (2) / Ln (1 + 12%)
- Doubling Time = 6.12 years
So if you choose investment in A, your funds will double in 7.27 years, but B will double more in 6.11 years.
Explanation
Although doubling time or the rule of 70 gives us the estimate of time to double our investment, the major assumption here is the constant growth rate. So if it is not constant, our estimate will be prone to errors and inaccurate. This happens in real life since interest rates do not remain constant and vary with time. So this concept is more of a theoretical concept and has less relevance in practical life. Another thing to remember is the interest rate in the doubling time in the rate per period. So if the compounding is happening monthly, we need to convert that rate into the monthly rate and then calculate the doubling time. But nevertheless, it is an important tool that helps us understand the compounding effect and is also very helpful in quickly working around to see the time it will take to double your money.
Relevance and Uses of Doubling Time Formula
Since the doubling formula will help you determine the time to double your investment, it also helps you to make decisions based on that. For example, if you know that, given the market rate, you will not be able to double your money in the time you want, you need to try to increase the growth rate by taking more risk and changing your portfolio allocation. But some limitations limit the use of the doubling formula. So, in a nutshell, This tool can only be used where the growth rate is expected to be steady and constant throughout the investment period, and if that rate is expected to vary, there is no point in using this formula.
Doubling Time Formula Calculator
You can use the following Doubling Time Calculator
| r | |
| Doubling Time Formula | |
| Doubling Time Formula | = |
| |||||
| = |
|
Recommended Articles
This has been a guide to Doubling Time Formula. Here we discuss How to Calculate Doubling Time along with practical examples. We also provide Doubling Time Calculator with a downloadable Excel template. You may also look at the following articles to learn more –