Updated July 28, 2023
Compounded Annual Growth Rate Formula (Table of Contents)
What is the Compounded Annual Growth Rate Formula?
Compounding is the effect where an investment earns interest not only on the principal component but also gives interest on interest. So compounded annual growth rate is the effective annual growth earned on investment considering compounding into the picture.
This assumes that the interest earned every year is reinvested and earns the same interest as the principal amount. That is why the compounded annual growth rate is always higher than the simple interest rate. Many investments like mutual funds and stock market returns are not very linear and in a very unstable fashion.
The compounded annual growth rate smooths out the return and indicates how much an investor has earned over the investment term, assuming all the earnings within that period are reinvested at the same rate. Because of this smoothing effect, it helps us compare data sets with different levels of volatility. It is very frequently used for the purpose of financial analysis.
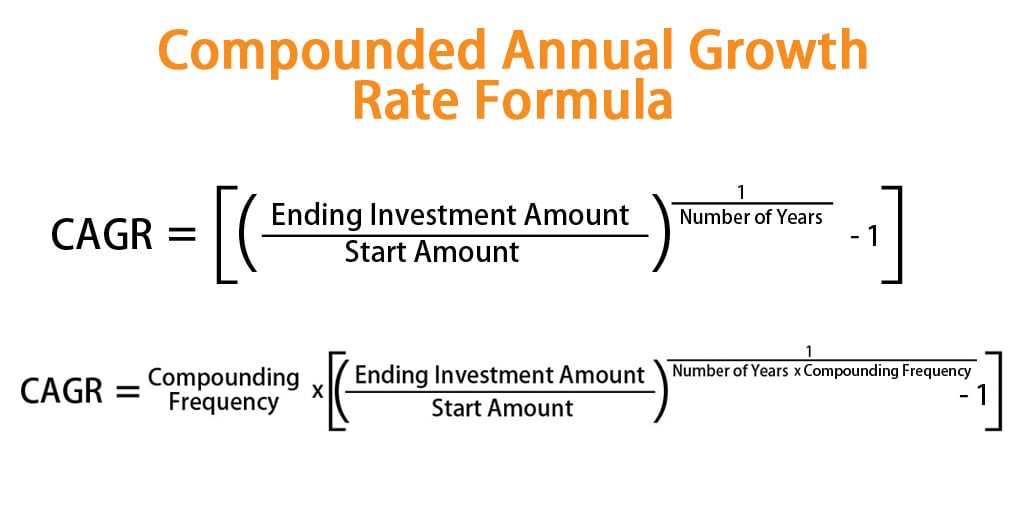
The formula for Compounded Annual Growth Rate –
This formula is applicable if the investment is compounded annually, which means that we are reinvesting the money annually. But sometimes, we want to calculate the rate where the compounding is happening quarterly, monthly, or daily. So for that, we use the below formula:
So, a formula for Compounded Annual Growth Rate –
Compounding Frequency:
- Semiannual Compounding: 2
- Quarterly Compounding: 4
- Monthly frequency: 12 and so on
Examples of CAGR Formula (With Excel Template)
Let’s take an example to understand the calculation of the CAGR Formula in a better manner.
CAGR Formula – Example #1
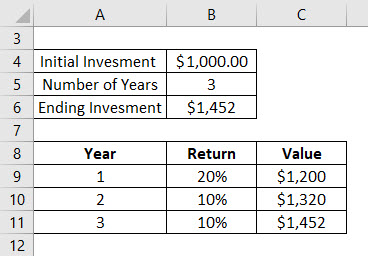
Let’s say you invested $1000 in mutual funds 3 years ago. Following is the return which you have got for these 3 years:
- 1st year, you got a 20% increase in value. So the total value is $1200 at the end of 1st year
- 2nd year, you got a 10% increase in value. So the total value is $1320 at the end of 2nd year
- 3rd year, you got a 10% increase in value. So the total value is $1452 at the end of 3rd year
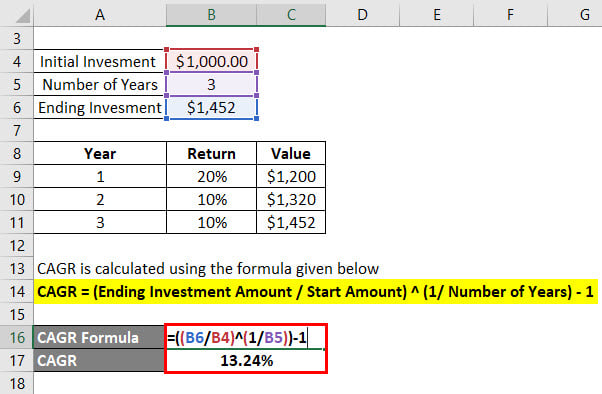
The formula to calculate CAGR is as below:
CAGR = (Ending Investment Amount / Start Amount) ^ (1/ Number of Years) – 1
- CAGR = ($1,452 / $1,000) ^ (1 / 3) – 1
- CAGR = 13.24%
The annual return for all three years varies, but the compounded annual growth rate provides a single rate that allows us to compare different investment opportunities.
CAGR Formula – Example #2
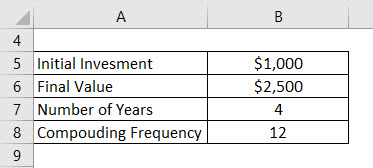
Let’s say you have invested $1000 in the bank and want to keep the money in the bank for 4 years. Now let’s say the total amount you get after 4 years is $2500. A bank is offering a rate with monthly compounding. Calculate CAGR.
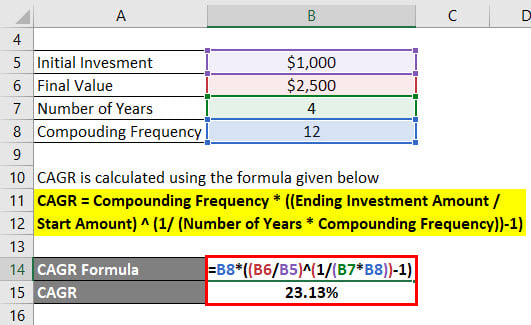
The formula to calculate CAGR is as below:
CAGR = Compounding Frequency * ((Ending Investment Amount / Start Amount) ^ (1/ (Number of Years * Compounding Frequency)) – 1)
- CAGR = 12 * (($2,500 / $1,000) ^ (1 / (4*12)) – 1)
- CAGR = 23.13%
So compounded annual growth rate is 23.13%.
Explanation of Compounded Annual Growth Rate Formula
Although the compound annual growth rate is the annual rate for the investment, it is only a theoretical figure and is not the true return. The major assumption here is that all the earnings are getting reinvested at the same rate for the investment period, but the rate will not remain for all the years, and we may not invest our money at the same rate. So it is only the representative rate that tells us what we might end up with if all the money is reinvested at that rate at the end of each year. So there are some key points that we should take into consideration while using compounded annual growth rate.
Also, we will be careful about an investment that is long in period. For example, if an investment period is very long, say 20 years, the compounded annual interest rate might give us the wrong indication because it can happen that we are not earning any profits during the first 15 years, and all the returns are coming in the last period. Earning no profits for 15 years is unacceptable for any business to sustain.
Similarly, suppose two investment opportunities have the same CAGR. In that case, it can be the case that one is more attracted than the other because growth in one is happening in the initial period while for the other, it is concentrated at the end of the period.
Relevance and Uses of Compounded Annual Growth Rate Formula
The compound annual growth rate is helpful in calculating the average growth rate of the investment and can help in comparing different investments. As we have seen in the above example, the year-to-year growth of investment is uneven and erratic. But using compounded annual growth rate, the return smoothens out. Another factor that makes compounded annual growth rate a critical method in determining an investment’s growth is that it considers the compounding effect, which the annual return rate doesn’t. Compounded annual rate does not give us the actual picture of the return since it only calculates the return on the principal amount and ignores the interest on the interest component. However, this is not the case with the compounded annual growth rate.
Compounded Annual Growth Rate Formula Calculator
You can use the following Compounded Annual Growth Rate Calculator
| Ending Investment Amount | |
| Start Amount | |
| No. of Years | |
| CAGR | |
| CAGR = | [(Ending Investment Amount / Start Amount) 1 /No. of Years-1] |
| = | [(0 / 0)1 / 0-1] = 0 |
Recommended Articles
This has been a guide to Compounded Annual Growth Rate formula. Here we discuss how to calculate CAGR along with practical examples. We also provide Compounded Annual Growth Rate calculator with a downloadable Excel template. You may also look at the following articles to learn more –