Updated July 27, 2023
Coupon Bond Formula (Table of Contents)
What is Coupon Bond Formula?
The term “coupon” refers to the periodic interest payment received by bondholders, and bonds that make such payments are known as coupon bonds.
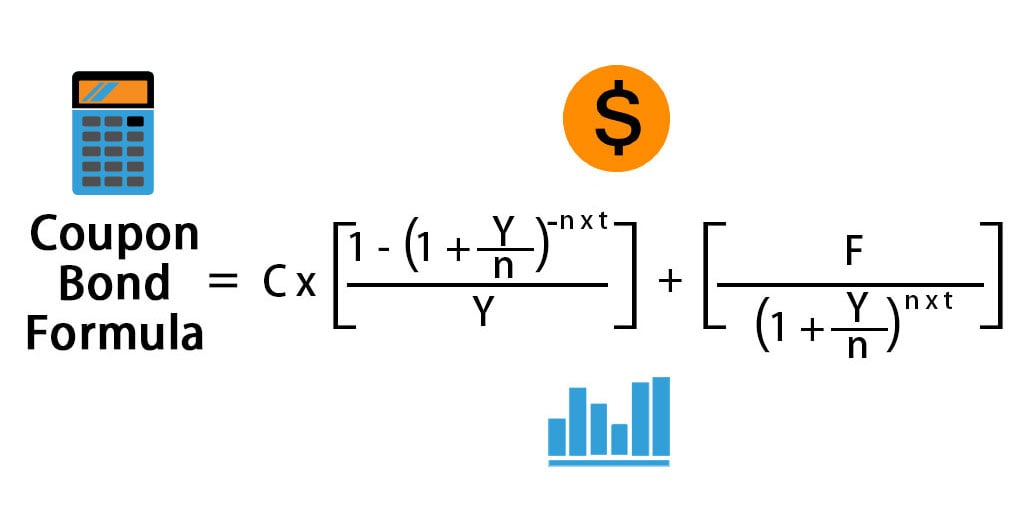
The formula for a coupon bond determines the bond’s price by discounting the probable future cash flows to present value and then summing up all of them. It expresses the coupon as a percentage of the bond’s par value. The future cash flows are the periodic coupon payments and the par value to be received at maturity. Mathematically, we represent the formula for a coupon bond as:
or
where,
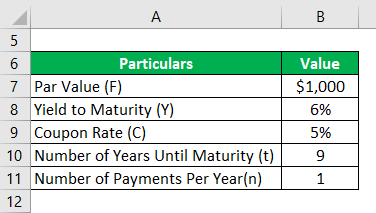
- C = Annual Coupon Payment,
- F = Par Value at Maturity,
- Y = Yield to Maturity,
- n = Number of Payments Per Year
- t = Number of Years Until Maturity
Examples of Coupon Bond Formula (With Excel Template)
Let’s take an example to understand the calculation of Coupon Bonds in a better manner.
Coupon Bond Formula – Example #1
Let us take the example of some coupon-paying bonds issued by DAC Ltd. One year back, the company had raised $50,000 by issuing 50,000 bonds worth $1,000 each. The bonds offer a coupon rate of 5% to be paid annually, and the bonds have a maturity of 10 years i.e. 9 years until maturity. As per the current market trend, the bonds with similar risk profiles have yielded to maturity of 6%. Calculate the market price of the bonds based on the given information.
Solution:
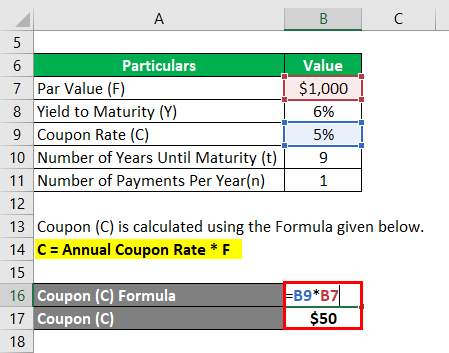
The formula to calculate Coupon (C) is as below:
C = Annual Coupon Rate * F
- C = 5% * $1000
- C = $50
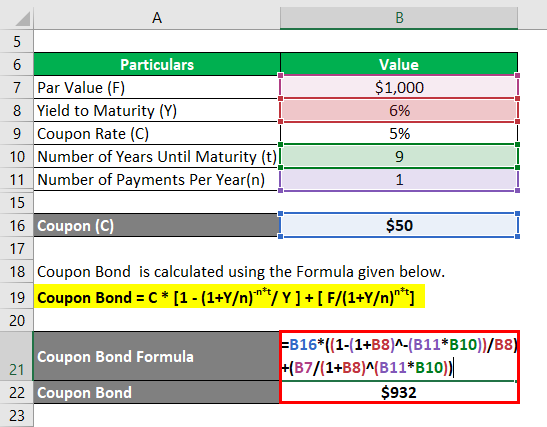
The formula to calculate Coupon Bond is as below:
Coupon Bond = C * [1 – (1+Y/n)-n*t/ Y ] + [ F/(1+Y/n)n*t]
- Coupon Bond = $50 * [1 – (1 + 6%/1) -1*9] + [$1000 / (1 + 6%/1)1*9
- Coupon Bond = $932
Therefore, the current market price of each coupon bond is $932, which means it is traded at a discount (current market price lower than par value).
Coupon Bond Formula – Example #2
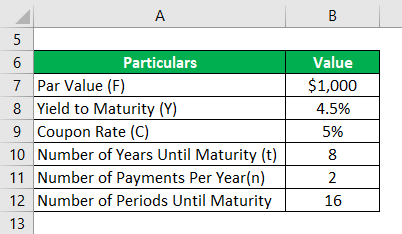
Let us take the same example mentioned above. In this case, the coupon rate is 5% but is to be paid semi-annually, while the yield to maturity is currently at 4.5%. Two years have passed since bond issuance; as such, are 8 years left until maturity. Calculate the market price of the bonds based on the new information.
Solution:
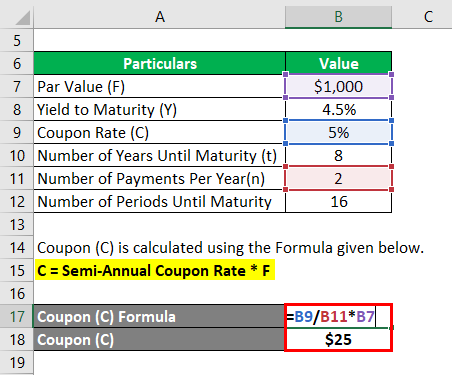
The formula to calculate Coupon (C) is as below:
C = Annual Coupon Rate * F
- C =(5%/2) * $1000
- C = $25
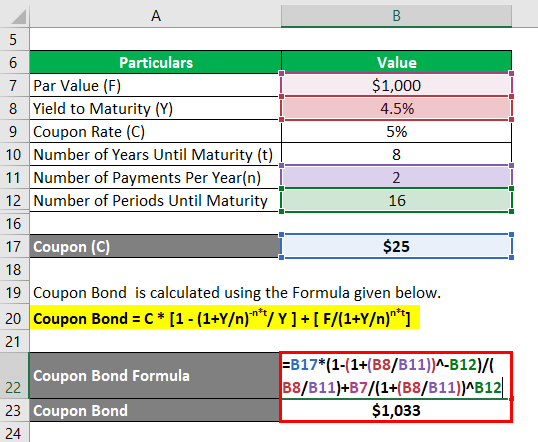
The formula to calculate Coupon Bond is as below:
Coupon Bond = C * [1 – (1+Y/n)-n*t/ Y ] + [ F/(1+Y/n)n*t]
- Coupon Bond = $25 * [1 – (1 + 4.5%/2)-16] + [$1000 / (1 + 4.5%/2)16
- Coupon Bond = $1,033
Therefore, the current market price of each coupon bond is $1,033, which means it is traded at a premium (current market price higher than par value).
Explanation
The formula for coupon bond can be derived by using the following steps:
Step 1: First, figure out the par value of the bond being issued and it does not change over the course of its tenure. It is denoted by F.
Step 2: Next, figure out the rate of the annual coupon and, based on that, calculate the periodic coupon payment of the bond. The coupon payment is the product of the coupon rate and the bond’s par value. It also does not change over the course of the bond tenure. The annual coupon payment is denoted by C and mathematically represented below.
Step 3: Next, figure out the total number of years until maturity of the bond, and it is denoted by t.
Step 4: Next, figure out the bond yield to maturity based on the current return expected from securities with similar levels of risk. Y denotes the yield to maturity.
Step 5: Next, figure out the number of periodic payments made during the year, and it is denoted by n. It is analogous to the number of compounding per year.
Step 6: Next, discount all the future coupon payments and par value using the yield to maturity to calculate the respective present value (PV).
PV of 1st Periodic Coupon = (C/n) /(1+Y/n)1
PV of 2nd Periodic Coupon = (C/n) /(1+Y/n)2
………..
PV of last Periodic Coupon = (C/n) /(1+Y/n)n*t
PV of Par Value = F/(1+Y/n)n*t
Step 7: Finally, the formula for coupon bonds can be derived by summing up the present value of all the coupon payments and the par value, as shown below.
Bond Price = ∑ [(C/n) /(1+Y/n)i] +[ F/(1+Y/n)n*t]
Relevance and Uses
From the perspective of investors or companies, it is important to understand the concept of pricing coupon bonds because they are a significant funding source in the capital market. If a bond offers a lower coupon rate than the market, the bond investor intends to bring down its price so its return matches the market return. Inherently, investors are attracted to bonds with higher coupon rates. So, as more and more investors purchase these high-yield bonds and push the prices up, it eventually brings its return to the level of the market. This is how the bond market works.
Recommended Articles
This has been a guide to Coupon Bond Formula. We discuss calculating Coupon Bonds, practical examples, and a downloadable Excel template here. You may also look at the following articles to learn more –