Updated July 26, 2023
Real Interest Rate Formula (Table of Contents)
What is the Real Interest Rate Formula?
The term “real interest rate” refers to the interest rate that has been adjusted by removing the effect of inflation from the nominal interest rate. In other words, it is effectively the actual cost of debt for the borrower or actual yield for the lender.
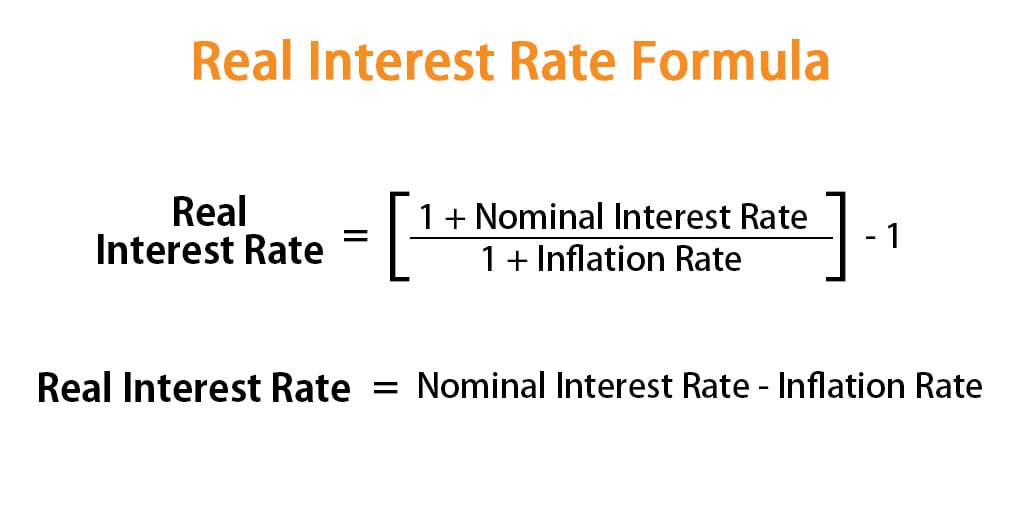
The formula for real interest rate can be derived by dividing one plus the nominal interest rate by one plus the inflation rate during the period. Mathematically, it is represented as,
On the other hand, the formula for real interest rate can be approximated as per Fisher equation and be expressed as the difference between the nominal interest rate and the inflation rate during the period. Mathematically, it is represented as,
Examples of Real Interest Rate Formula (With Excel Template)
Let’s take an example to understand the calculation of Real Interest Rate in a better manner.
Example #1
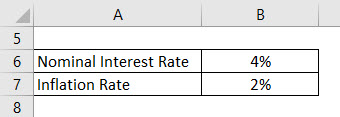
Let us take the example of David who has recently invested a sum of $20,000 in a long term deposit fund. The tenure of the fund is 10 years and the annualized nominal interest rate offered is 4%. If the inflation rate during the period is expected to be 2%, then calculate the real interest rate as per the full formula and the approximate formula.
Solution:
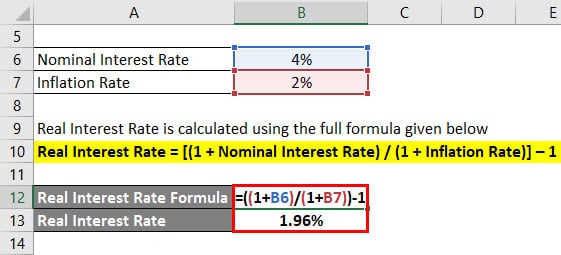
Real Interest Rate is calculated using the full formula given below
Real Interest Rate = [(1 + Nominal Interest Rate) / (1 + Inflation Rate)] – 1
- Real Interest Rate = [(1 + 4%) / (1 + 2%)] – 1
- Real Interest Rate = 1.96%
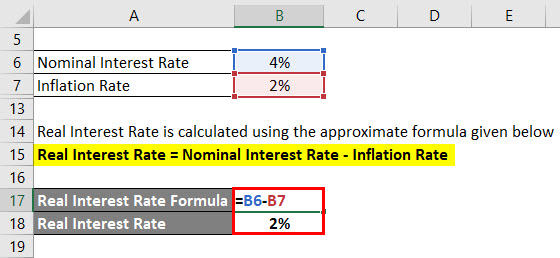
Real Interest Rate is calculated using the approximate formula given below
Real Interest Rate = Nominal Interest Rate – Inflation Rate
- Real Interest Rate = 4% – 2%
- Real Interest Rate = 2%
Therefore, the real interest is expected to be 1.96% and 2% according to full and approximate formula respectively.
Example #2
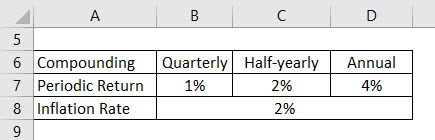
Let us take the example of John who is in the process of making an investment decision. The bank has offered him three interest rate plans –
- Quarterly Interest Rate of 1%,
- Half-Yearly Interest Rate of 2% and
- Annual Interest Rate of 4%.
Now, help John to decide which plan will offer him the best real interest rate if the inflation rate during the period is expected to be 2%.
Solution:
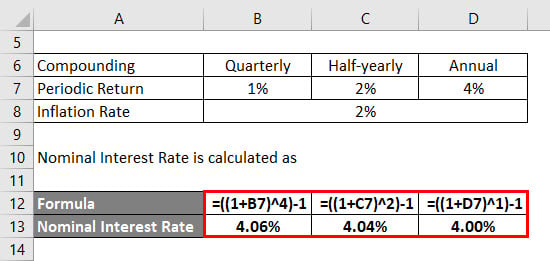
Nominal Interest Rate is calculated as
For Quarterly
- Nominal Interest Rate = [(1 + 1% )4 – 1]
- Nominal Interest Rate = 4.06%
For Half-Yearly
- Nominal Interest Rate = [(1 + 2% )2 – 1]
- Nominal Interest Rate = 4.04%
For Annual
- Nominal Interest Rate = [(1 + 4% )1 – 1]
- Nominal Interest Rate = 4.00%
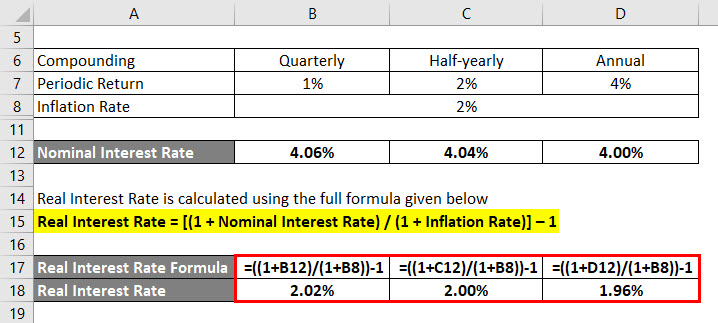
Real Interest Rate is calculated using the full formula given below
Real Interest Rate = [(1 + Nominal Interest Rate) / (1 + Inflation Rate)] – 1
For Quarterly
- Real Interest Rate = [(1 + 4.06%) / (1 + 2%)] – 1
- Real Interest Rate = 2.02%
For Half-Yearly
- Real Interest Rate = [(1 + 4.04%) / (1 + 2%)] – 1
- Real Interest Rate = 2.00%
For Annual
- Real Interest Rate = [(1 + 4.00%) / (1 + 2%)] – 1
- Real Interest Rate = 1.96%
From the above results, it can be clearly seen that the 1st option with a quarterly return of 1% will offer the best real interest rate of return.
Explanation
The formula for Real Interest Rate can be derived by using the following steps:
Step 1: Firstly, determine the nominal interest rate which is usually an annual rate of interest documented for any given investment.
Step 2: Next, determine the inflation rate during the period. Usually, the inflation rate is annualized and it can be easily available from various government databases, such as the U.S. Bureau of Labor Statistics. In fact, Treasury Inflation-Protected Securities (TIPS) can be a good indicator of inflation rate in the US.
Step 3: Finally, The formula for real interest rate can be derived by dividing one plus the nominal interest rate (step 1) by one plus the inflation rate (step 2) during the period as shown below.
Real Interest Rate = [(1 + Nominal Interest Rate) / (1 + Inflation Rate)] – 1
On the other hand, according to the Fisher equation, the formula for the real interest rate can be derived by deducting the inflation rate during the period from the nominal interest rate as shown below.
Real Interest Rate = Nominal Interest Rate – Inflation Rate
Relevance and Uses of Real Interest Rate Formula
From an investor’s perspective, it is important to understand the concept of real interest rate because it captures the real growth of the wealth after adjusting the inflation rate. One needs to be cognizant of the fact that inflation erodes the value of every stream of cash flows, either mainstream like salary or passive like return on investment. As such, it is essential that we consider the impact of inflation while making a decision about any investment from which we expect a stream of cash flows in the future.
Real Interest Rate Formula Calculator
You can use the following Real Interest Rate Calculator
| Nominal Interest Rate | |
| Inflation Rate | |
| Real Interest Rate | |
| Real Interest Rate | = |
|
||||||||
| = |
|
Recommended Articles
This is a guide to Real Interest Rate Formula. Here we discuss How to Calculate Real Interest Rate along with practical examples. We also provide a Real Interest Rate Calculator with downloadable excel template. You may also look at the following articles to learn more –