Updated May 29, 2023
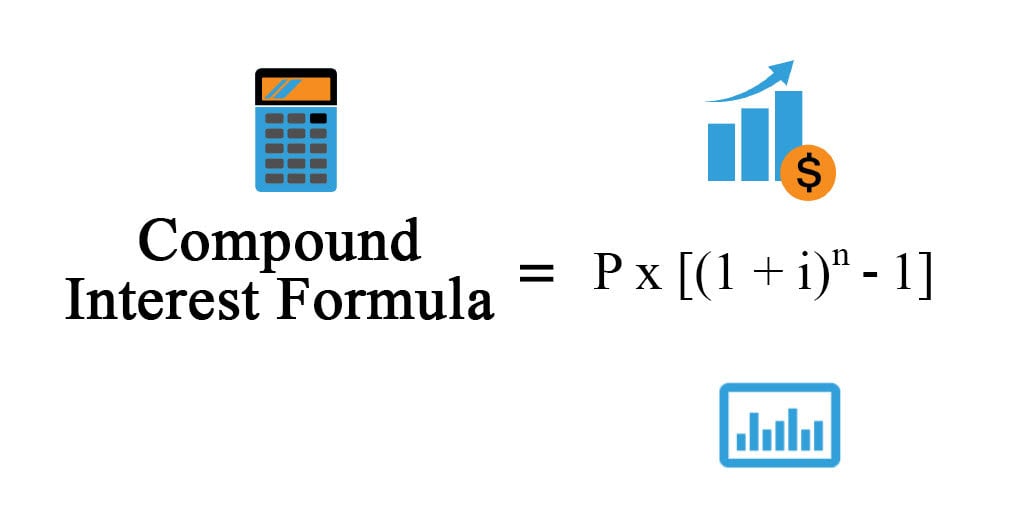
Compound Interest Formula (Table of Contents)
- Compound Interest Formula
- Examples of Compound Interest Formula (With Excel Template)
- Compound Interest Formula Calculator
Compound Interest Formula
Compound interest is the type of interest calculated on the initial principal, including all the interest accumulated in the prior periods of a loan or a deposit. This implies that interest earned in each period adds to the initial amount, so the next period’s interest calculation is based on the new, increased balance. As a result, the interest earned over time can be much higher than simple interest, which only calculates interest on the initial amount.
The formula for computing Compound Interests is:
Where,
- P = Initial Principal
- i = Interest Rate
- n = Number of compounding periods, which could be daily, annually, semi-annually, monthly, or quarterly
Explanation
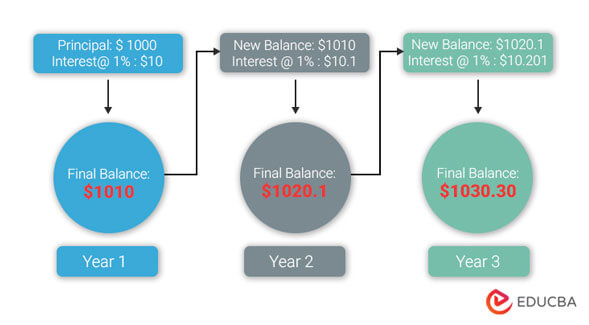
To understand how compound interest works, let’s consider an example.
- Let’s say you deposit $1,000 in a savings account that earns an annual interest rate of 1%.
- At the end of the first year, you will have earned $10 in interest, for a total of $1,010.
- If you leave your money in the account and earn 1% interest the next year, you will earn interest on both your original $1,000 deposit and the $10 interest you earned in the previous year.
- This means that at the end of the second year, you will have earned $10.10 in interest, for a total of $1,020.10.
- If you continue to leave the money in the account and earn interest for several years, the amount of interest you earn each year will continue to grow as the accumulated interest grows.
- For example, after 3 years, the final balance would be $1,030.30. And, after 10 years, you would have earned a total of $105.17 in interest, for a total of $1,051.17.
The key to compound interest is time. The longer you leave your money invested and earn compound interest, the more your money will grow.
Examples of Compound Interest Formula (With Excel Template)
Let’s take an example to understand the calculation of Compound Interest in a better manner.
Example #1
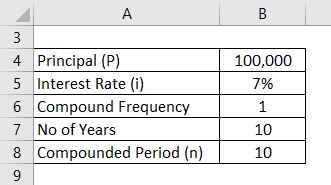
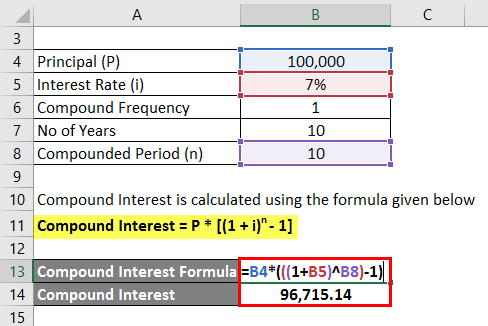
Mr. A deposited 100,000 in an FD, where the bank pays a 7% annual interest rate compounded annually. He wants to calculate the compound interest he will receive if he remains invested for 10 years.
Solution:
Compound Interest is calculated using the formula given below.
Compound Interest = P * [(1 + i)n – 1]
- Compound Interest = 100,000 * ((1 + 7%)10 – 1)
- Compound Interest = 96,715.14
Example #2
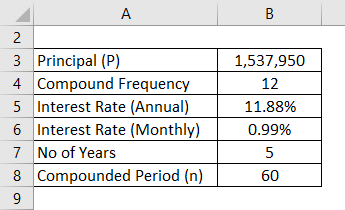
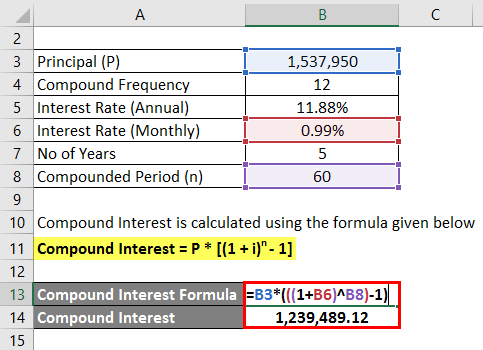
Vardhan is planning to buy a new car and wants to take out a loan for the remaining amount, with an initial down payment of 10,00,000. A bank has offered him a loan at a monthly compounded interest rate of 11.88%. Vardhan wants to pay off the loan in 5 years and needs to know the extra amount he will have to pay.
To calculate the compound interest for 5 years, we can use the variables given: P is 15,37,950 (25,37,950 -10,00,000), the Rate of interest is 11.88% divided by 12 which is 0.0099, i.e., 0.99%, n is 5 and frequency is 12 as its annually.
Solution:
Compound Interest = P * [(1 + i)n – 1]
- Compound Interest = 1,537,950 * ((1 + 0.99%)60 – 1) = 1,239,489.12
Vardhan would be paying an excessive amount of around 12 lakhs, the accumulated interest since he is making only the loan and principal payments at the end of the 5-year loan period.
Example #3
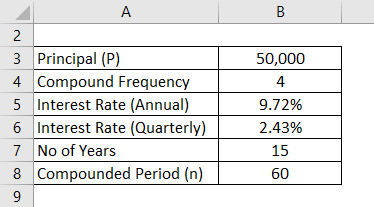
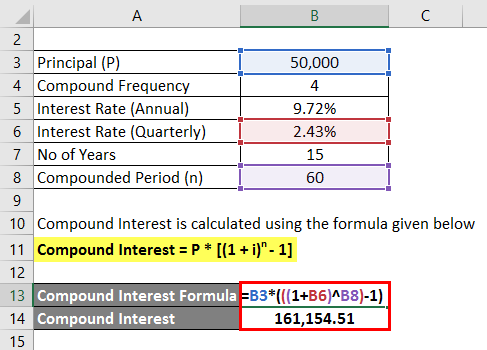
Invest Corp has launched a new investment product that has piqued Shankar’s interest. To invest in this scheme, Shankar needs to put in an initial amount of 50,000, and the investment will mature after 15 years. The guaranteed Rate of interest is 9.72%, which is tax-free, and the product also provides a bonus at the end of 15 years. Assume quarterly interest compound frequency. You are required to compute the total income earned in this product assuming Shankar decides to invest in the same, and at the end of 15 years, the bonus income is 10,783.90.
We are given all the variables here: P is 50,000, Rate of interest is 9.72% divided by 4, which is 0.0243, i.e., 2.43%, n is 15, and frequency is 4 as its paying quarterly.
Solution:
Compound Interest = P * [(1 + i)n – 1]
- Compound Interest = 50,000 * ((1 + 2.43%)60 – 1) = 161,154.51
The income earned on this product will be 161,154.51 + 10,783.90, equal to 171,938.41.
Explanation:
To compute compound interest, we need to follow the below steps:
- Step 1: Find out the initial principal amount required to be invested.
- Step 2: Divide the Rate of interest by several compounding periods if the product doesn’t pay interest annually. Compounding frequency could be 1 for annual, 2 for semi-annual, 4 for quarterly, and 12 for monthly.
- Step 3: Compound the interest for the Number of years and as per the compounding frequency.
- Step 4: Now, subtract 1 from the result in step 3 and then multiply it by the principal amount. This calculation yields the interest that would either be earned if the amount is invested or paid if a loan amount is taken.
Relevance and Uses
- Compound interest calculates the interest on the initial amount invested, known as the principal, and includes all the interest accumulated from prior periods of a loan or deposit.
- As mentioned earlier, you can calculate compound interest by multiplying the initial amount invested, the principal amount, by 1 plus the annual Rate of interest raised to the Number or frequency of compounding periods and then subtracting 1.
- When calculating compound interest, the Number or the frequency of compounding periods will make a big difference, or one can say a significant difference.
- Banks, capital markets, and stock markets primarily use these to estimate growth rates.
Compound Interest Formula calculator
You can use the following Compound Interest Calculator.
| P | |
| i | |
| n | |
| Compound Interest Formula | |
| Compound Interest Formula = | P x [(1 +i)n -1] |
| = | 0 x [(1 +0)0- 1] = 0 |
Frequently Asked Questions (FAQs)
Q1. What are the types of compound interest?
Answer: There are generally two types of compound interest: annual compounding and continuous compounding. In annual compounding, the interest is calculated and added to the principal once a year (usually at the end of each year). The interest is calculated and added to the principal constantly throughout the year in continuous compounding.
Q2. What are the applications of the compound interest formula?
Answer: People use the compound interest formula in a wide range of applications, including:
- Personal finance (calculating the returns on savings accounts, bonds, and mutual funds and determining the total cost of borrowing money, such as loans and credit cards)
- Business finance (calculating the future value of investments and determining the interest payments on loans)
- Economics (modeling the effects of inflation and calculating the present value of future cash flows)
- Mathematics (using it in a variety of applications in pure mathematics, such as calculus and number theory)
- Science (modeling the growth of populations, the accumulation of radioactive decay, and the dynamics of chemical reactions)
Q3. Where is compound interest used in real life?
Answer: Savings accounts, loans, investments, and retirement savings all use compound interest in real life. The bank pays you interest on your balance for savings accounts, usually compounded daily, monthly, or annually. In the case of loans, lenders add interest to the principal balance each month, which can significantly increase the total repayment amount. Compound interest also benefits investments and retirement savings, as reinvesting the returns can lead to significant growth over time.
Q4. What is the Rule of 72?
Answer: The Rule of 72 is a quick mental calculation to estimate the time it takes for an investment or debt to double in value or size by dividing the number 72 by the annual interest rate or growth rate.
For example, an investment with a 7.2% annual interest rate will double in value in approximately 10 years (72/7.2 = 10).
Recommended Articles
This is a guide to Compound Interest Formula. Here we discussed how to Calculate Compound Interest along with practical examples. We also have provided Compound Interest Calculator with a downloadable Excel template. You may also look at the following articles to learn more –