Updated July 26, 2023
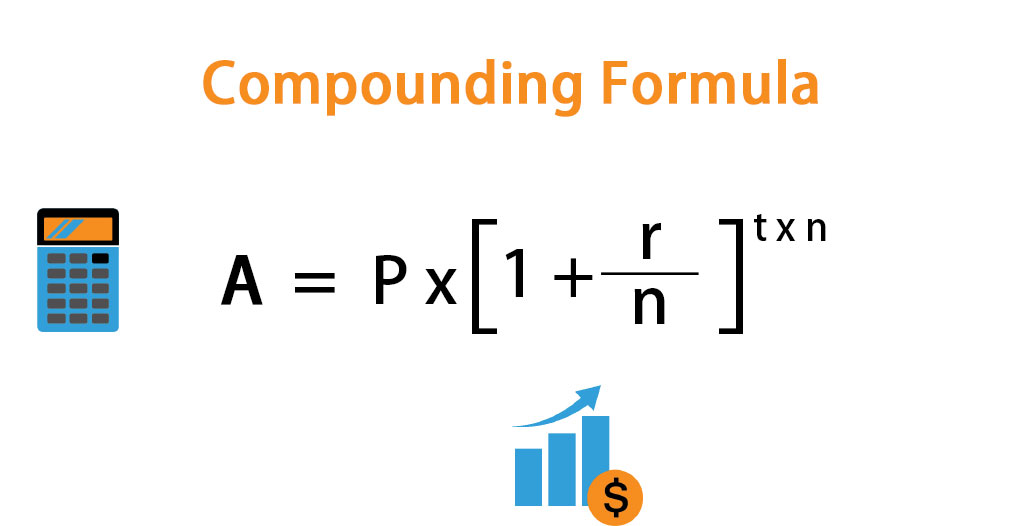
Compounding Formula (Table of Contents)
What is a Compounding Formula?
The term “compounding” refers to the accumulation of wealth based on growth in both principal and interest earned in the previous periods.
The formula for compounding involves a calculation of the compounded amount, which can be derived on the basis of initial amount, interest rate, tenure, and frequency of compounding per year. Mathematically, it is represented as,
Where,
- A = Compounded Amount
- P = Initial Amount
- r = Interest Rate
- t = Tenure
- n = Frequency of Compounding Per Year
Further, the formula for continuous compounding is slightly different, requiring initial amount, interest rate, and tenure. Mathematically, it is represented as,
Examples of Compounding Formula (With Excel Template)
Let’s take an example to understand the calculation of Compounding in a better manner.
Compounding Formula – Example #1
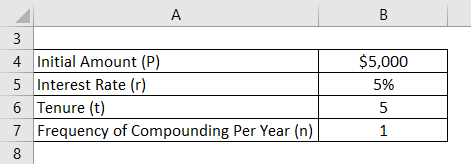
Let us take the example of a sum of $5,000 that has been deposited for 5 years at an interest rate of 5% to be compounded annually. Then, calculate the compounded amount at maturity.
Solution:
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n
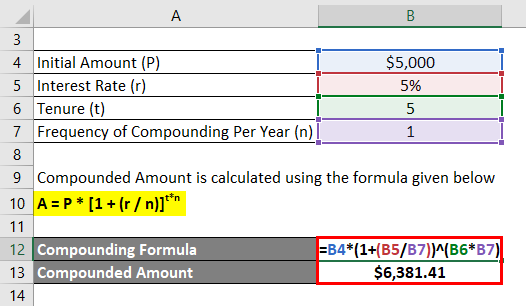
- Compounded Amount = $5,000 * (1 + (5%/1))5*1
- Compounded Amount = $6,381.41
Therefore, as a result of the compounding effect, the amount is expected to grow upto $6,381.41 at the time of maturity.
Compounding Formula – Example #2
Let us take the example of David, who has decided to deposit a lump sum amount of $1,000 in the bank for 5 years. Now, he has recently learned about the effect of compounding on the final amount at the time of maturity and seeks to calculate the same for his deposited sum. Help David in calculating the compounding effect if the interest rate is 4% and the compounding is done:
- Annually
- Half Yearly
- Quarterly
- Monthly
- Daily
- Continuous

Solution:
For Annually
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n
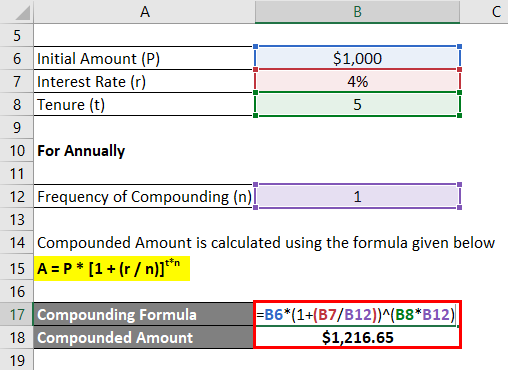
- Compounded Amount = $1,000 * (1 + (4%/1))5*1
- Compounded Amount = $1,216.65
For Half Yearly
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n
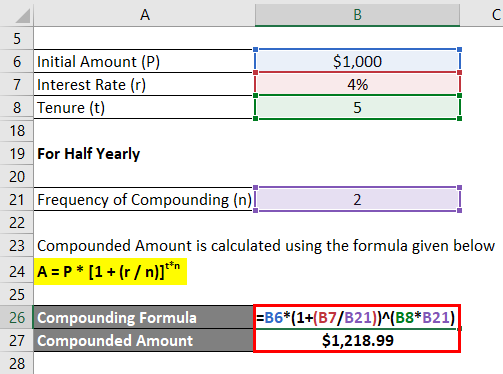
- Compounded Amount = $1,000 * (1 + (4%/2))5*2
- Compounded Amount = $1,218.99
For Quarterly
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n
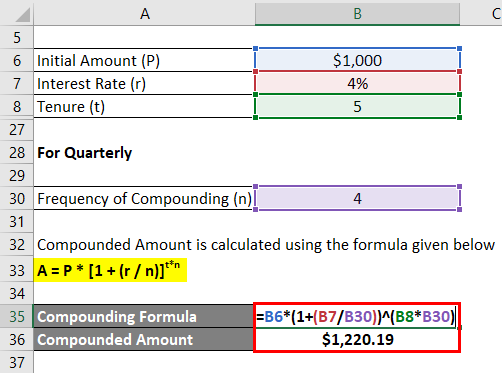
- Compounded Amount = $1,000 * (1 + (4%/4))5*4
- Compounded Amount = $1,220.19
For Monthly
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n
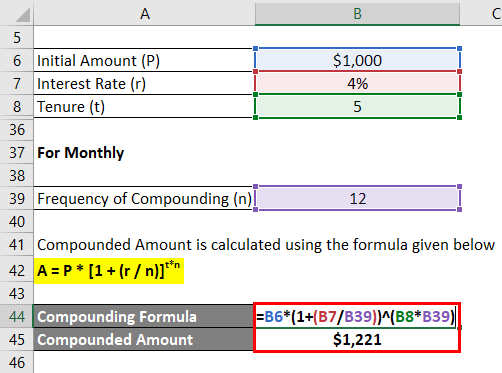
- Compounded Amount = $1,000 * (1 + (4%/12))5*12
- Compounded Amount = $1,221.00
For Daily
Compounded Amount is calculated using the formula given below
A = P * [1 + (r / n)]t*n

- Compounded Amount = $1,000 * (1 + (4%/365))5*365
- Compounded Amount = $1,221.39
For Continuous
Compounded Amount for continuous compounding is calculated using the formula given below.
A = P * er*t
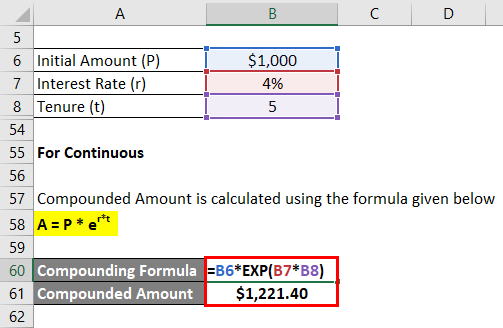
- Compounded Amount = $1,000 * e4%*5
- Compounded Amount = $1,221.40
From the above results, it can be concluded that the compounding effect leads to an increase in the final amount with the increase in the frequency of compounding per year.
Explanation
The formula for compounding can be derived by using the following simple steps:
Step 1: Firstly, figure out the initial amount that is usually the opening balance of a deposit or loan. It is denoted by ‘P’.
Step 2: Next, figure out the interest rate that is to be charged on the given deposit or loan. It is denoted by ‘r’.
Step 3: Next, figure out the tenure, which is the number of years remaining till the maturity. It is denoted by ‘t’.
Step 4: Next, figure out the frequency of compounding in a year, and it is denoted by ‘n’.
Step 5: Finally, the formula for compounded amount can be derived by using the initial amount (step 1), interest rate (step 2), tenure (step 3), and frequency of compounding per year (step 4) as shown below.
A = P * (1 + r/n)t*n
On the other hand, the formula for continuous compounding is expressed using the initial amount (step 1), interest rate (step 2) and tenure (step 3), as shown below.
A = P * er*t
Relevance and Uses of Compounding Formula
It is important to understand the concept of compounding as it helps to comprehend how a certain sum of money can multiply with the change in the frequency of compounding. In fact, the rate at which the final amount accumulates depends on the frequency of compounding, such that the final amount increases with the increase in the frequency of compounding during a period. The compounding effect finds application across the financial system where it is used to compute the maturity amount. However, the frequency of compounding can vary from case to case, such as savings account at a bank usually uses daily compounding, while CDs use daily, monthly or semi-annual compounding.
Compounding Formula Calculator
You can use the following Compounding Calculator
| P | |
| r | |
| t | |
| n | |
| A | |
| A = P * [1 + r / n]t*n |
| 0 * [1 + 0 / 0]0*0 = 0 |
Recommended Articles
This is a guide to Compounding Formula. Here we discuss How to Calculate Compounding along with practical examples. We also provide a Compounding Calculator with a downloadable excel template. You may also look at the following articles to learn more –


