Updated November 18, 2023

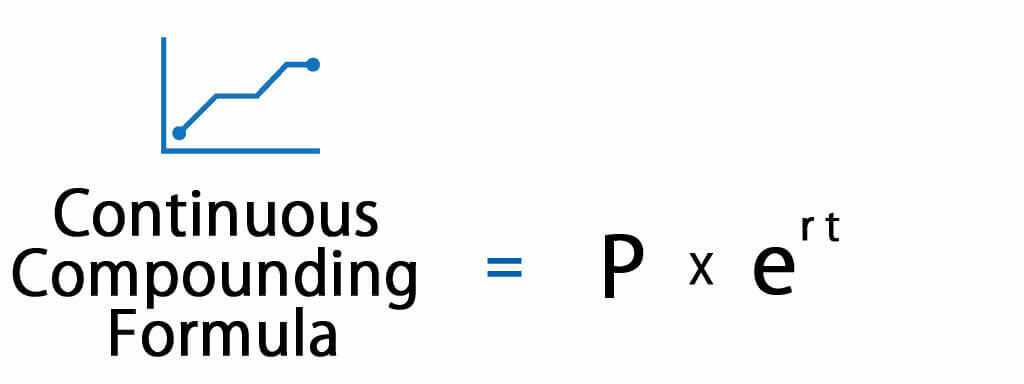
Continuous Compounding Formula

Let’s first understand compounding interest before jumping to the continuous compounding concept.

Compounding interest means the interest investors earn each year is added to their principal so that the amount doesn’t only grow, it grows at an increasing rate than the simple interest rate – it is one of the most useful financial concepts.

It is the basis of everything from a long-term investment plan in the share market to a personal savings plan. It also considers the effects of inflation on the amount and the importance of paying debtOverhead Ratio Formula | Calculator (Excel template).

For continuously compounding interest rate gets added on every moment. This makes calculation tough. Financial institutions do not use this for interest rate charges as there is little difference in the continuous and daily compounding amounts. Banks use daily compounding interest amounts in some of their products.

The formula for continuous compounding is as follows:

The continuous compounding formula calculates the interest earned, which is continuously compounded for an infinite period.

where,

P = Principal amount (Present Value of the amount)
t = Time (Time is years)
r = Rate of Interest.

The above calculation assumes constant compounding interest over an infinite time period. As the time period mentioned is infinite, the exponent function (e) helps multiply the current investment amount. This is multiplied by the current interest rate and time period. Despite many investments, the difference between total interest earned through continuous compounding in Excel is the same as traditional compounding interest.

Examples & Explanation of Continuous Compounding Formula

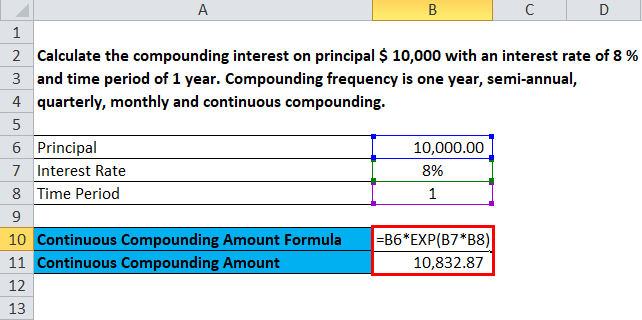
Calculate the compounding interest on the principal of $ 10,000 with an interest rate of 8 % and a time period of 1 year. Compounding frequency is one-year, semi-annual, quarterly, monthly, and continuous compounding.

You can download this Continuous Compounding Template here – Continuous Compounding Template

Annual Compounding Future Value:

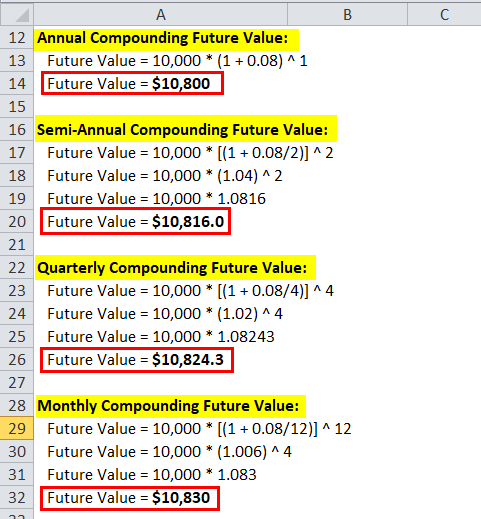
Future Value = 10,000 * (1 + 0.08) ^ 1
Future Value = $10,800

Semi-Annual Compounding Future Value:

Future Value = 10,000 * [(1 + 0.08/2)] ^ 2
Future Value = 10,000 * (1.04) ^ 2
Future Value = 10,000 * 1.0816
Future Value = $10,816.0

Quarterly Compounding Future Value:

Future Value = 10,000 * [(1 + 0.08/4)] ^ 4
Future Value = 10,000 * (1.02) ^ 4
Future Value = 10,000 * 1.08243
Future Value = $10,824.3

Monthly Compounding Future Value:

Future Value = 10,000 * [(1 + 0.08/12)] ^ 12
Future Value = 10,000 * (1.006) ^ 4
Future Value = 10,000 * 1.083
Future Value = $10,830

Continuous Compounding Future Value:

Future Value = 10,000 * e ^0.08
Future Value = 10,000 * 1.08328
Future Value = $10,832.87

As can be seen from the above example of compounding calculations with different frequencies, the interest calculated from continuous compounding is $832.9, which is only $2.9 more than monthly compounding. So it makes the case of using monthly or daily compounding interest rates in practical life rather than continuous compounding interest rates.

Significance and Use

The importance of continuous compounding formula is:

Instead of continuous compounding of interest on an annual, quarterly, or monthly basis, continuous compounding Excel will efficiently reinvest gains over perpetually.
The continuous compounding effect allows the compounding of interest amount to be reinvested at the same interest rate, thereby allowing an investor to earn returns exponentially.
Continuous compounding determines that it is not only the principal amount that will earn money, but the continuous compounding of interest amount will also keep multiplying the amount.

Compound interest occurs annually, semi-annually, quarterly, daily, or continuously. In this process, the interest earned at the end of each time period becomes the new principal. In semi-annual compounding, the financial institution adds interest to the principal every six months, and this cycle continues until maturity. Similarly, in annual compounding, the institution adds interest annually; in quarterly compounding, it adds interest every three months, and in daily compounding, interest is added the following day.

Continuous Compounding Calculator

You can use the following Continuous Compounding Calculator

P
r
t
Continuous Compounding Formula =

Continuous Compounding Formula =	P x e^{(r x t)}
=	0 x e^{(0 x 0)} = 0

Continuous Compounding Formula in Excel (With Excel Template)

Here we will do the same example of the Continuous Compounding formula in Excel. It is very easy and simple. You need to provide the three inputs i.e. Principal amount, Rate of Interest, and Time.

You can easily calculate the Continuous Compounding using Formula in the template provided.

First, we need to calculate the Continuous Compounding amount using a Formula