Updated October 7, 2023
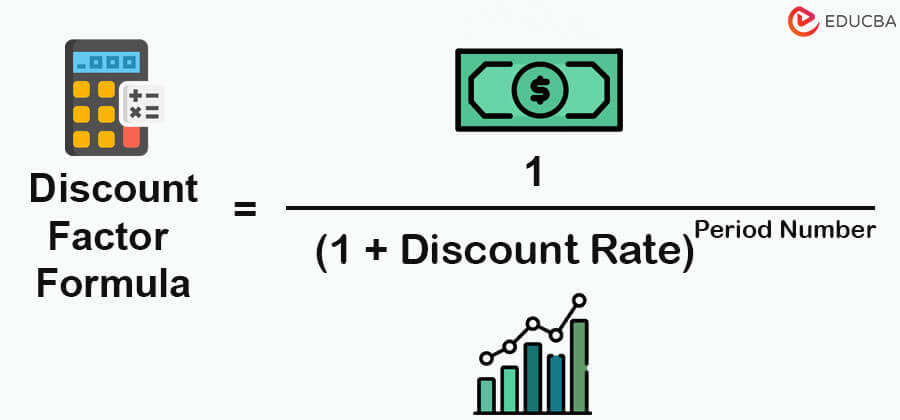
Table of Contents
- Discount Factor Formula – Introduction
- How to Calculate It?
- Examples of Discount Factor Formula (With Excel Template)
- Calculator
Discount Factor Formula – Introduction
The discount factor formula helps us find the net present value (NPV) of future cash flows, meaning it finds how much a future cash flow is worth in today’s terms.
It is an important formula used in financial modeling for calculating DCF (Discounted Cash Flow). It determines the current value of future investments.
The discount factor formula is:
Where,
- Discount Rate: It is the expected rate of return on an investment.
- Period Number: The period (year or month) from which the future cash flow is.
How to Calculate the Discount Factor?
Follow the below steps to calculate the discount factor.
- Determine the discount rate (r)
- Determine the future period number (n) at which you want to calculate the discount factor.
- Add 1 to r
- Raise this calculated value to the power of n
- Finally, divide by 1.
Examples of Discount Factor Formula (With Excel Template)
Let’s understand the calculation of the discount factor formula from the following examples.
Discount Factor Formula – Example #1
In this example, we will calculate the discount factor when the discount rate is 10% and the period is 2 years.

Solution:
The formula to calculate the discount factor is as follows:
Discount Factor = 1 / (1 + Discount Rate)^Period Number
= 1 / (1 + 10%) ^ 2) = 0.83
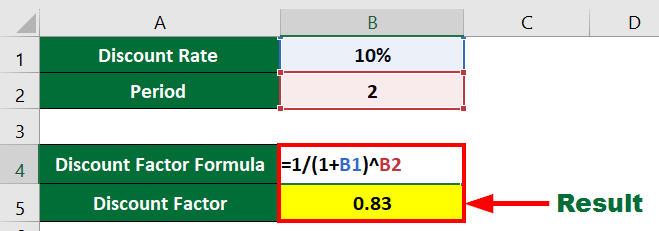
Result: The discount factor for the discount rate of 10% for 2 years is 0.83.
Discount Factor Formula – Example #2
In this example, we will calculate the discount factor for continuous compounding when the discount rate is 12% and the duration is two years. Here, we need to calculate at different intervals like:
- Daily
- Monthly
- Quarterly
- Half-yearly
Solution:
1. Daily
The formula to calculate the discount factor for daily compounding is,
Discount Factor = 1 / (1 + Discount Rate/365)^(365 x Period Number)
=1 / (1 + 4% / 365) ^ (365 x 2) = 0.9231

2. Monthly
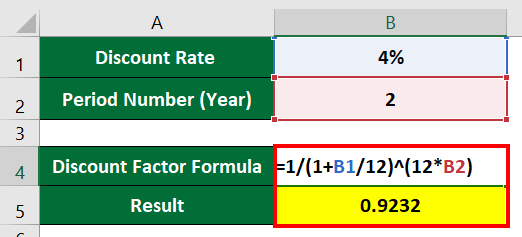
The formula to calculate the discount factor for monthly compounding is,
Discount Factor = 1 / (1 + Discount Rate/12)^(12 x Period Number)
=1 / (1 + 4% / 12) ^ (12 x 2) = 0.9232
3. Quarterly
The formula to calculate the discount factor for quarterly compounding is as follows.
Discount Factor = 1 / (1 + Discount Rate/4)^(4 x Period Number)
=1 / ( 1 + 4% / 4) ^ (4 x 2) = 0.9235
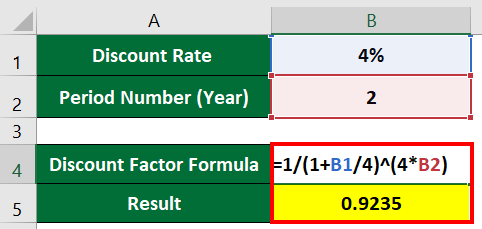
4. Half Yearly
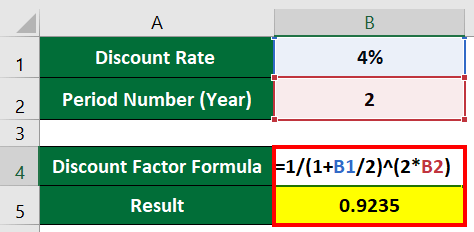
The formula to calculate the half-yearly compounding discount factor is as follows.
Discount Factor = 1 / (1 + Discount Rate/2)^(2 x Period Number)
=1 / (1 + 4% / 2) ^ (2 x 2) = 0.9238
Discount Factor Formula – Example #3
In the above example, we have only calculated the discount factor. Now, we will learn to calculate the net present value from the discount factor.
Suppose a company generates $100,000 undiscounted cash flow for 7 years. Now, we need to calculate the present value for each year if the annual discount rate is 8% and the total period is 7 years.

Solution:
Step 1: First, we will calculate the discount factor for each year with the following formula.
Discount Factor = 1 / (1 + Discount Rate)^Period Number
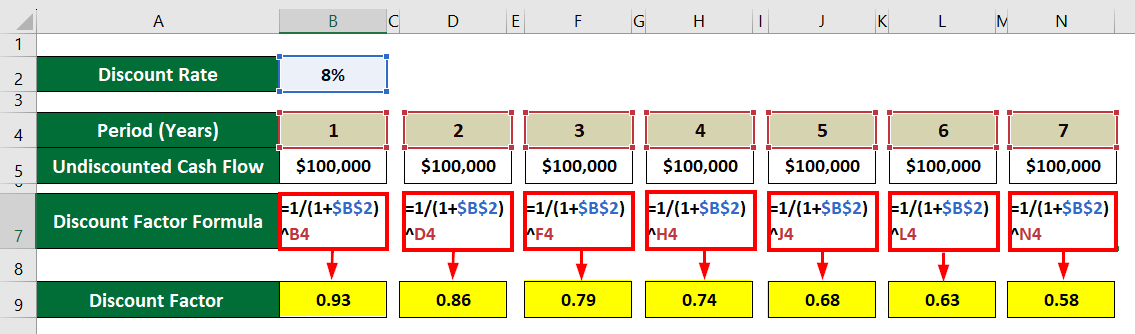
Calculation:
- Discount factor for 1st year = 1 / (1 + 8%) ^ 1) = 0.93
- Discount factor for 2nd year = 1 / (1 + 8%) ^ 2) = 0.86
- Discount factor for 3rd year = 1 / (1 + 8%) ^ 3) = 0.79
- Discount factor for 4th year = 1 / (1 + 8%) ^ 4) = 0.74
- Discount factor for 5th year = 1 / (1 + 8%) ^ 5) = 0.68
- Discount factor for 6th year = 1 / (1 + 8%) ^ 6) = 0.63
- Discount factor for 7th year = 1 / (1 + 8%) ^ 7) = 0.58
Step 2: Now, let us calculate each year’s present value with the formula below.
Present Value = Undiscounted Cash Flow x Discount Factor

Calculation:
- Present Value for 1st year = $100,000 x 0.93 = $92,592.6
- Present Value for 2nd year = $100,000 x 0.86 =$85,733.9
- Present Value for 3rd year = $100,000 x 0.79 = $79,383.2
- Present Value for 4th year = $100,000 x 0.74 = $73,503.0
- Present Value for 5th year = $100,000 x 0.68= $68,058.3
- Present Value for 6th year = $100,000 x 0.63 = $63,017.0
- Present Value for 7th year = $100,000 x 0.58 = $58,349.0
Step 3: Now, we will calculate the net present value.
Net Present Value = Sum of Present Value of all 7 years
= $92,592.6 + $85,733.9 + $79,383.2 + $73,503 + $68,058.3 + $63,017 + $58,349
= $520,637
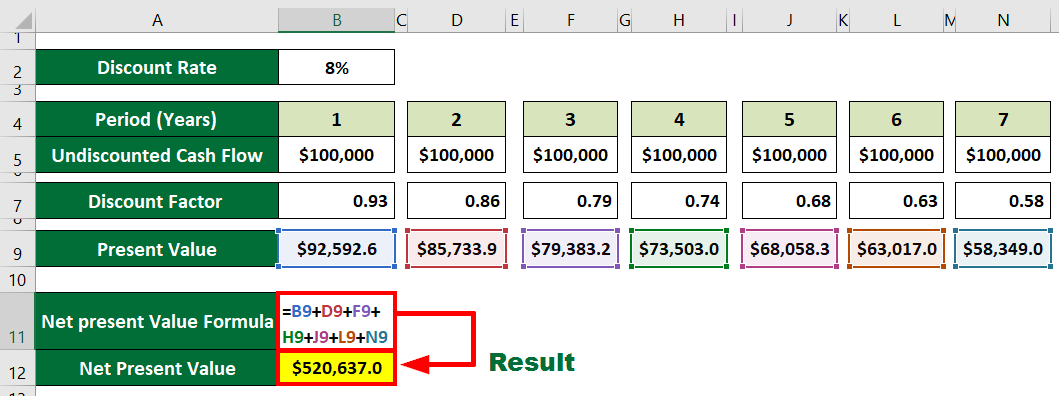
Result: The net present value of the company with $100,000 undiscounted cash flows, considering an 8% discount rate over 7 years, is $520,637.
Discount Factor Formula – Example #4
In this example, we will calculate the net present value manually as well as using the XNPV Excel function. In addition, we need to determine the cumulative year, i.e., the period number between the given start and end date.
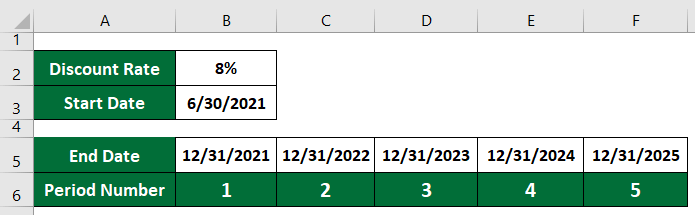
Solution:
Step 1: First, we will calculate the number of days from the start to the end date with the below formula.
No. of days = End Date – Start Date
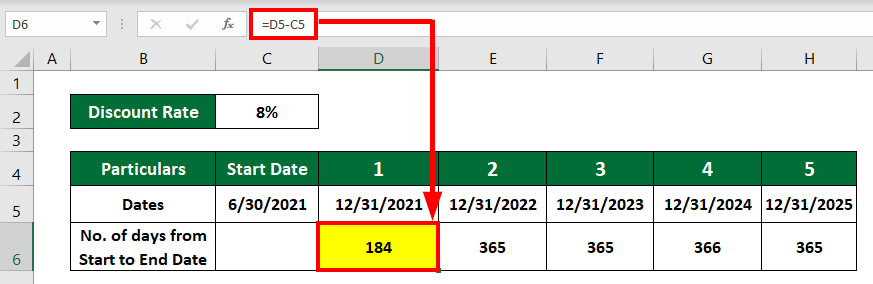
Calculation:
- Period 1: 12/31/2021 – 6/30/2021 = 184
- Period 2: 12/31/2022 – 12/31/2021 = 365
- Period 3: 12/31/2023 – 12/31/2022 = 365
- Period 4: 12/31/2024 – 12/31/2023 = 366
- Period 5: 12/31/2025 – 12/31/2024 = 365
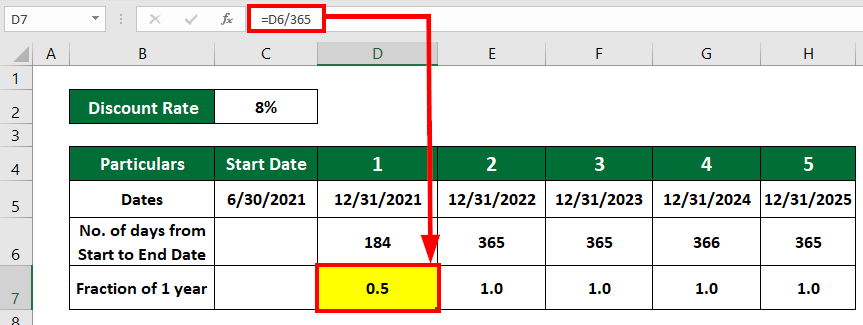
Step 2: Here, we calculate the fraction of 1 year using the following formula.
Fraction of year = Total no of days from start to end date/ 365
Step 3: Let’s calculate the cumulative year, i.e., the total number of periods.
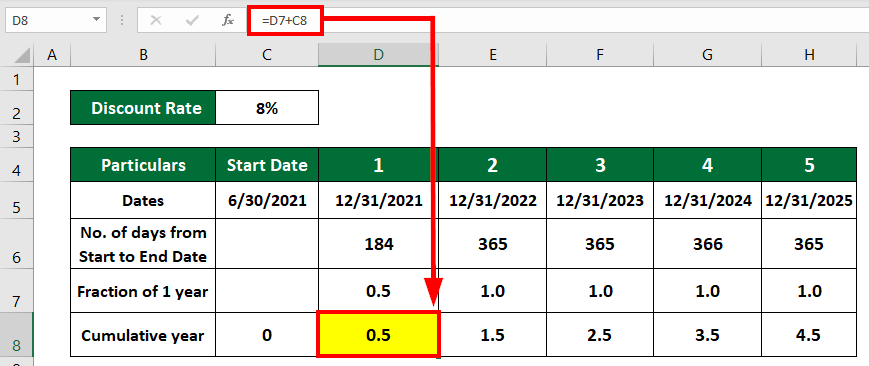
Calculation:
- Period 1: 0.5 + 0 = 0.5
- Period 2: 1.0 + 0.5 = 1.5
- Period 3: 1.0 + 1.5 = 2.5
- Period 4: 1.0 +2.5 = 3.5
- Period 5: 1.0 + 3.5 = 4.5
Step 4: Now, to calculate the discount factor for each period, we use the formula given below.
Discount Factor = 1 / (1 + Discount Rate)^Period Number
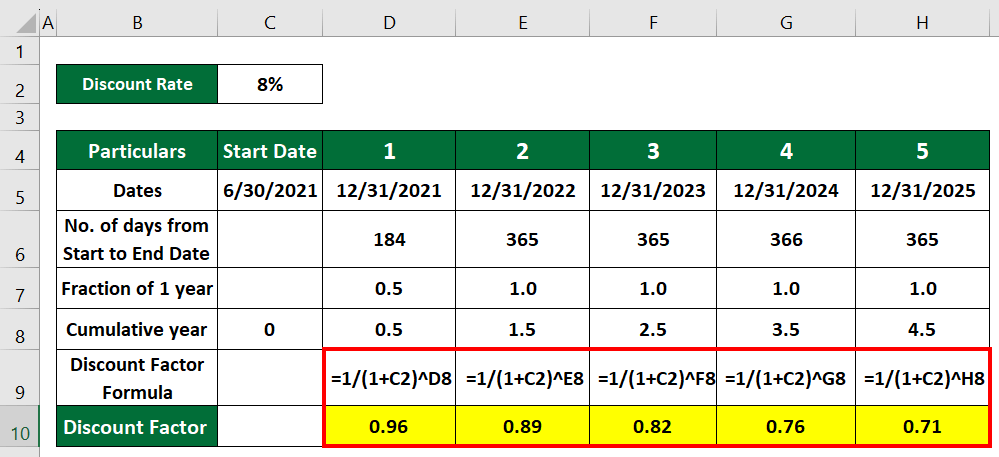
Calculation:
- Discount factor for Period 1 = 1 / (1 + 8%) ^ 0.5)= 0.96
- Discount factor for Period 2 = 1 / (1 + 8%) ^ 1.5) = 0.89
- Discount factor for Period 3 = 1 / (1 + 8%) ^ 2.5) = 0.82
- Discount factor for Period 4 = 1 / (1 + 8%) ^ 3.5) = 0.76
- Discount factor for Period 5= 1 / (1 + 8%) ^ 4.5) = 0.71
Step 5: Let’s calculate the present value for each period.
Present Value = Undiscounted Cash Flow x Discount Factor
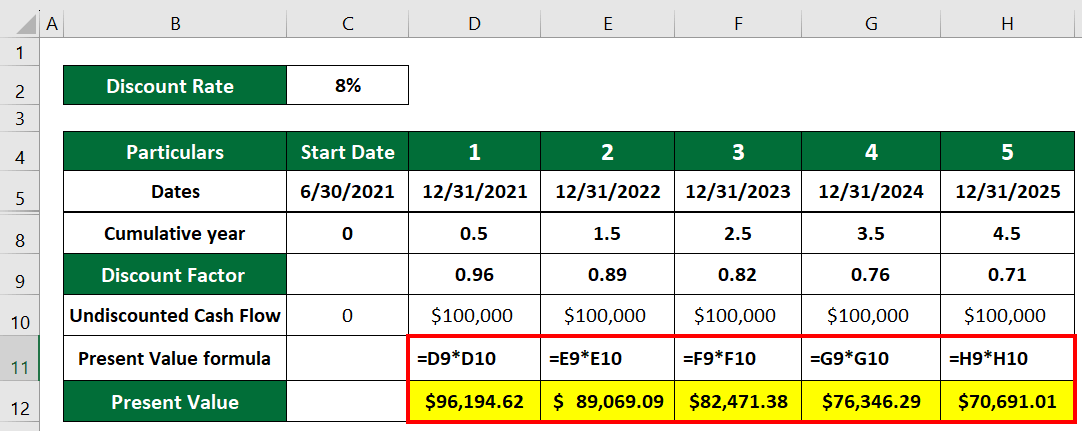
Calculation:
- Present value for Period 1 = $100,000 x 0.96 = $96,194.62
- Present value for Period 2= $100,000 x 0.89 = $89,069.09
- Present value for Period 3 = $100,000 x 0.82 = $82,471.38
- Present value for Period 4 = $100,000 x 0.76 = $76,346.29
- Present value for Period 5 = $100,000 x 0.71 = $70.691.01
Step 6: Let’s calculate the net present value using the formula given below:
Net Present Value = Sum of Present Value of each period
= $96,194.62 + $89,069.09 + $82,471.38 + $76,346.29 + $70.691.01
= $414,772.38

Now, we will calculate the net present value using the following XNPV formula:

According to our data, it will be
=XNPV(Discount Rate, Sum of Undiscounted Cash Flow, Sum of Time Period)
In the screenshot below, we have shown the cells that you must select while applying the XNPV formula.

Result: The XNPV formula shows a net present value of $414,772.38, which matches our manual calculation.
Calculator
You can use the following discount factor formula calculator.
| Discount Rate | |
| Period Number | |
| Discount Factor Formula = | |
| Discount Factor Formula = |
| |||||||||
|
Frequently Asked Questions (FAQs)
Q1. Why is the discount factor important?
Answer: The discount factor is important for calculating future cash flows’ present value or current worth. It is also important for the following reasons.
- This process allows for assessing project profitability and can be particularly helpful in making investment decisions, such as capital budgeting.
- It also lets a company evaluate risks and calculate the value of investments with higher risk.
- It also lets us determine the valuation of financial assets, including bonds.
- The technique is critical in financial modeling, DCF analysis, pension plans, and insurance companies for discounting their liabilities.
Q2. How to determine a discount rate?
Answer: Calculating a discount rate is complex and depends on various financial and investment-specific factors. These factors include the risk-free rate, market conditions, project-specific factors, the required rate of return, the cost of capital, and regulatory and accounting considerations. Companies usually determine the discount rate using the cost of debt and equity, i.e., the weighted average cost of capital (WACC).
Q3. What does a discount factor of less than 1 indicate?
Answer: When the discount factor is less than 1, it indicates that the future cash flows are worth less in present value due to the time value of money. It means that a sum to be paid or received in the future is worth less in today’s terms. For example, a dollar you get today is worth more than the one you get in the future.
Recommended Articles
This article is a complete guide on how to calculate the discount factor using the discount factor formula. You may also look at the following articles to learn more –

