Updated July 21, 2023
Definition of Effective Duration
Effective duration measures the change in the price of a bond to a 1% or a 100 basis point change in the yield of the bond across all maturities and therefore a parallel shift of the yield curve by 1% indicating the amount of interest rate risk the bondholder needs to bear by holding the given bond in his investment portfolio.
Modified duration is more appropriate for straight bonds i.e. those bonds which don’t have any embedded options as modified duration assumes that the cash flows of the bond remain constant. However, this is not always the case because bonds with embedded options such as callable or putable bonds have cash flows that may change over the life of the bond.
For such bonds, it is better to use effective duration, which is also known as the option-adjusted duration of the curve duration.
Formula
- PV– = PV of cash flows when the yield goes down parallelly
- PV+ = PV of cash flows when the yield goes up parallelly
- PV0 = Current Price of the Bond
- ∆Curve = Amount of parallel shift in the yield curve
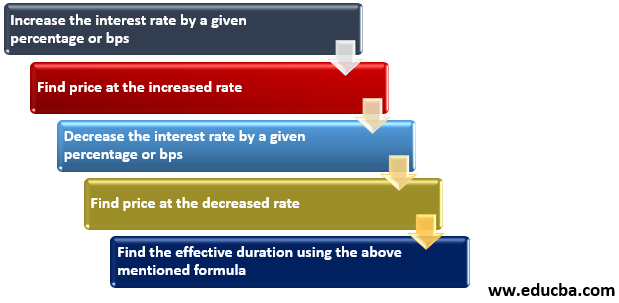
Process of Calculating the Effective Duration
The process of calculating the effective duration is given below:
- The cash flows of a bond with an embedded call option, callable at par would not be affected too much if the yield is way above the coupon rate and the change being tested ineffective duration is less than that required to bring it below the coupon rate.
- Similarly, the cash flows of a bond with an embedded put option, put able at par would not be affected too much if the yield is way below the coupon rate and the change being tested ineffective duration is less than that required to bring it above the coupon rate.
- In such circumstances, the bonds with embedded options behave like a straight bond.
Examples of Effective Duration (With Excel Template)
Let’s take an example to understand the calculation of Effective Duration in a better manner.
Example #1
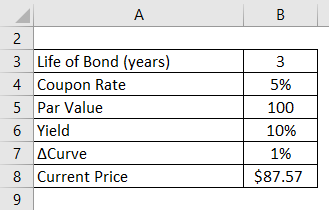
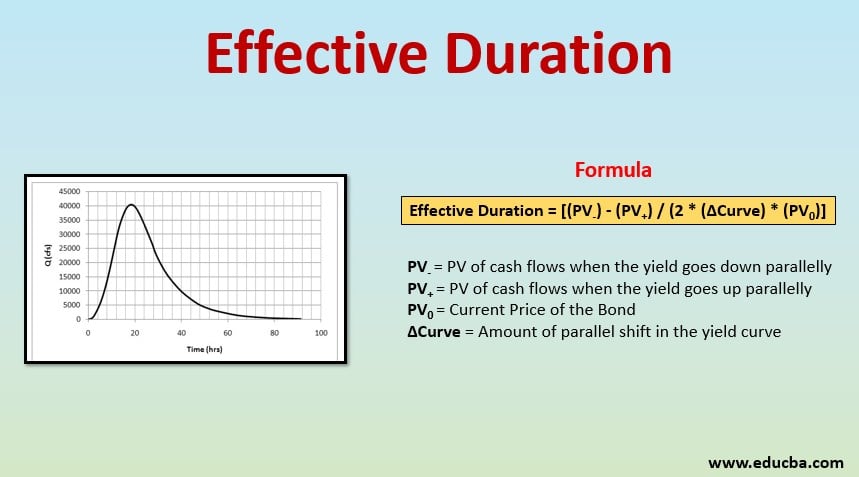
Suppose we are given the following information:
- Life of bond = 3 years
- Coupon rate = 5%
- Par value = $100
- Option = Callable at par in 1 year or 2 years from now
- Yield = 10%
- Current price = $87.57
- ∆Curve = 100bps or 1%
Calculate the Effective Duration
Solution:
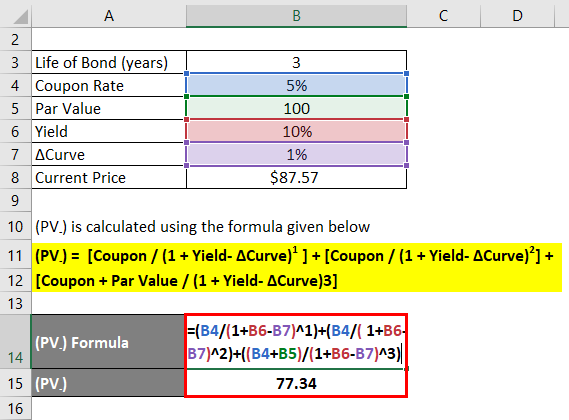
(PV–) is calculated using the formula given below
(PV–) = [Coupon / (1 + Yield- ∆Curve)1 ]+ [Coupon / (1 + Yield- ∆Curve)2]+ [Coupon + Par Value / (1 + Yield- ∆Curve)3]
- (PV–) = [5% / (1 + 10% – 1%)1 ]+ [ 5%/ (1 + 10% – 1%)2]+ [5% + 100 / (1 + 10% – 1%)3]
- (PV–) = 77.34
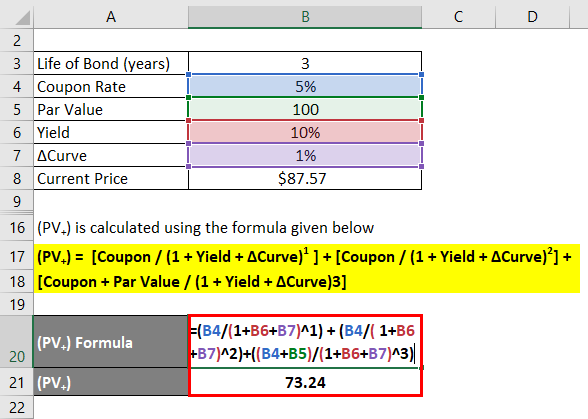
(PV+) is calculated using the formula given below
(PV+) = [Coupon / (1 + Yield + ∆Curve)1 ]+ [Coupon / (1 + Yield + ∆Curve)2]+ [Coupon + Par Value / (1 + Yield + ∆Curve)3]
- (PV+) = [5% / (1 + 10% + 1%)1 ]+ [ 5%/ (1 + 10% + 1%)2]+ [5% + 100 / (1 + 10% + 1%)3]
- (PV+) = 73.24
Effective Duration is calculated using the formula given below
Effective Duration = [(PV–) – (PV+) / (2 * (∆Curve) * (PV0)]
- Effective Duration = [(77.34) – (77.24) / (2 * (1%) * (87.57)]
- Effective Duration = 2.34
This implies that the change of 1% in the yield brings a change of 2.34% in the price of the bond. Therefore this is a measure of the interest rate sensitivity of the bond.
Benefits of Effective Duration
- Bonds with Embedded Option: Other measures of interest rate sensitivity such as Modified duration don’t tackle the bonds which can have a change in the cash flows due to option embedded nature. Effective duration is appropriate for such bonds.
- Comprehensive Approach: If we go deeper into the calculations of Effective duration, it uses the concepts of option-adjusted spread and interest rate volatility also, which is a much more comprehensive approach than other measures of interest rate sensitivity.
- Can be Used to Compare Bonds: If two bonds are identical in most respects but vary in some, the duration is a good measure to see which bond is more appropriate for the investment portfolio of the investor and which fits better within the risk & return objectives of the investment policy statement (IPS).
- Helpful in Planning Investment: The expected movement of the interest rate curve can be used and duration can be calculated in various scenarios to figure out the range of movement in the price of the bond. This can be used by investors to plan how much of their investment portfolio can go into the given investment.
Limitations of Effective Duration
- Ignores Convexity: As with all measures of duration, even effective duration is not so good a measure of estimating interest rate risk as the convexity of the price to yield curve is not captured in the same because the duration is only the first-order differential while the curvature of the curve can only be captured by the second-order differential that is measured by effective convexity.
- Difficult Calculation: If the bond is of a complex nature the calculation of effective duration can get highly complicated. Factoring in the option-adjusted spread and interest rate volatility leads to the development of binomial trees of cash flows of the bonds and which include several interim steps. So the calculation becomes even more complicated and therefore only highly skilled professionals may be able to calculate the same.
- Equal Movement in Either Direction: The assumption of increasing and decreasing the interest rate by the same amount, that is, 100 bps as shown in the example that we discussed above, is not very realistic because most of the time, this movement is skewed in one direction. Either the increase is greater or the decrease is greater, therefore the reliability of the measure is impacted and therefore the measure may not be a good predictor of actual movements in the bond prices in alignment with that of the interest rates.
- Only One Factor Considered: Factors other than the interest rate movement which also affect the price of the bond is not taken into consideration and so effective duration or as a matter of fact, and measure of duration can’t be used in isolation to measure the appropriateness of security for the investment portfolio.
Conclusion
Effective duration is the most comprehensive measure of duration, which can be used for a bond with or without the embedded options as it doesn’t assume the cash flows to remain constant over the life of the security. The inputs that go into the calculation address the issue of interest rate volatility and also consider the option-adjusted spread and so it does consider several factors that impact the magnitude of the movement of interest rates. However, the convexity of the price to yield curve is overlooked in the calculation and therefore the measure is only an approximation as are other measures of duration.
Recommended Articles
This is a guide to Effective Duration. Here we discuss how to calculate Effective Duration along with practical examples. We also provide a downloadable excel template. You may also look at the following articles to learn more –