Difference Between Geometric Mean vs Arithmetic Mean
The Arithmetic mean and Geometric mean are the tools widely used to calculate the returns on investment for investment portfolios in the world of finance. People use the arithmetic mean to report the higher returns which are not the correct measure of calculating the return on investment. Since the return on investment for a portfolio over the years is dependent on returns in previous years, the Geometric mean is the correct way to calculate the return on investment for a specific time period. The arithmetic mean is better suited in the situation wherein variables being used for the calculation of average are not dependent on each other.
Example: Suitability use of Geometric mean vs Arithmetic mean
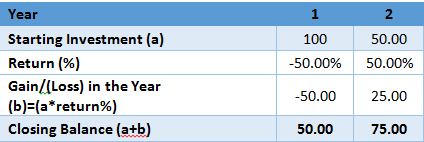
1. Let’s take an example of return on investment for an amount of $100 over 2 years. Suppose the returns in two years were -50% and +50% in the 1st and 2nd Average return calculation by using arithmetic mean will be 0% (Arithmetic mean = (-50%+50%) /2 = 0%)
This gives a wrong impression that the investor is breaking even on its investment, and there is no loss or profit. However, a closer analysis gives an entirely different picture of the scenario.
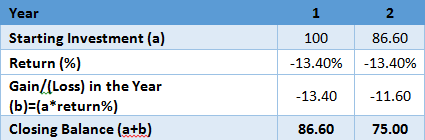
From the above table, we can see that the investment of $100 after -50% and +50% return in year 1 and 2, will be close to $75.Therefore, the investor is not breaking even on its investment as suggested by the arithmetic mean average, but he has incurred a loss of $25 after 2 years on its investment. This is well reflected by using Geometric mean to calculate the return on the investment over 2 years as below:
The geometric mean of returns
This means the annualized return on the portfolio had been negative at 13.40%. The investment position after two years is as below:
Therefore, the Geometric mean shows the true picture of investment that there is a loss in investment with an annualized negative return of -13.40%. Since the return in each year impacts the absolute return in the next year, a geometric mean is a better way to calculate the annualized return on investment.
2. When one needs to calculate the average of variables that are not dependent on each other, Arithmetic means a suitable tool to calculate the average. The average of marks of a student for 5 subjects can be calculated by the arithmetic mean as scores of the student in different subjects are independent of each other.
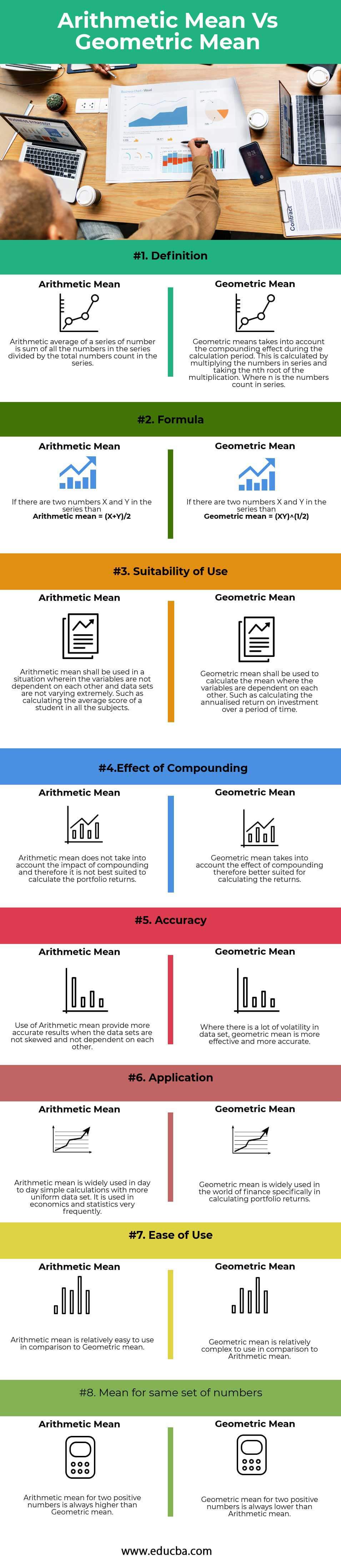
Head to Head Comparison between Geometric Mean vs Arithmetic Mean (Infographics)
Below is the top 8 difference between Geometric Mean vs Arithmetic Mean:
4.9 (3,296 ratings)
View Course
Key Differences between Geometric Mean vs Arithmetic Mean
Let us discuss some of the major differences between Geometric Mean vs Arithmetic Mean:
- Both Geometric Mean vs Arithmetic Mean are the tools to calculate the returns on investment in finance and also used in other applications such as economics, statistics.
- The arithmetic mean is calculated by dividing the sum of the numbers by number count. However, Geometric means take into account the compounding effect during the calculation.
- The geometric mean is the correct way to calculate the return on investment for a specific time period Since the returns on investment for a portfolio over the years are interdependent. However, the Arithmetic mean is better suited in the situation wherein variables being used for calculation are not dependent on each other.
- The arithmetic mean is more useful and accurate when it is used to calculate the average of a data set where numbers are not skewed and not dependent on each other. However, in the scenario where there is a lot of volatility in a data set, a geometric mean is more effective and more accurate.
- The arithmetic mean is relatively easier to calculate and use in comparison to the Geometric mean, which is relatively complex to calculate.
- The geometric mean is very widely used in the world of finance, specifically in the calculation of portfolio returns. However, an Arithmetic mean is not an appropriate tool to use in return calculation.
- The Arithmetic mean of two numbers is always higher than the Geometric mean of the same numbers.
Geometric Mean vs Arithmetic Mean Comparison Table
Let’s look at the top 8 Comparison between Geometric Mean vs Arithmetic Mean
| The Basis Of Comparison |
Arithmetic Mean |
Geometric Mean |
| Definition | The arithmetic average of a series of numbers is the sum of all the numbers in the series divided by the counts of the total number in the series. | Geometric means takes into account the compounding effect during the calculation period. This is calculated by multiplying the numbers in a series and taking the nth root of the multiplication. Where n is the numbers count in series. |
| Formula | If there are two numbers X and Y in the series than
Arithmetic mean = (X+Y)/2 |
If there are two numbers X and Y in the series than
Geometric mean = (XY)^(1/2) |
| Suitability of Use | Arithmetic means shall be used in a situation wherein the variables are not dependent on each other, and data sets are not varying extremely. Such as calculating the average score of a student in all the subjects. | Geometric mean shall be used to calculate the mean where the variables are dependent on each other. Such as calculating the annualized return on investment over a period of time. |
| Effect of Compounding | The arithmetic mean does not take into account the impact of compounding, and therefore, it is not best suited to calculate the portfolio returns. | The geometric mean takes into account the effect of compounding, therefore, better suited for calculating the returns. |
| Accuracy | The use of Arithmetic means to provide more accurate results when the data sets are not skewed and not dependent on each other. | Where there is a lot of volatility in the data set, a geometric mean is more effective and more accurate. |
| Application | The arithmetic mean is widely used in day to day simple calculations with a more uniform data set. It is used in economics and statistics very frequently. | The geometric mean is widely used in the world of finance, specifically in calculating portfolio returns. |
| Ease of Use | The arithmetic mean is relatively easy to use in comparison to the Geometric mean. | The geometric mean is relatively complex to use in comparison to the Arithmetic mean. |
| Mean for the same set of numbers | The arithmetic mean for two positive numbers is always higher than the Geometric mean. | The geometric mean for two positive numbers is always lower than the Arithmetic mean. |
Conclusion
Geometric Mean vs Arithmetic Mean both finds their application in economics, finance, statistics, etc. according to their suitability. The geometric mean is more suitable for calculating the mean and provides accurate results when the variables are dependent and widely skewed. However, an Arithmetic mean is used to calculate the average when the variables are not interdependent. Therefore, these two should be used in a relevant context to get the best results.
Recommended Articles
This has been a guide to the top difference between Geometric Mean vs Arithmetic Mean. Here we also discuss the Geometric Mean vs Arithmetic Mean key differences with infographics and comparison table. You may also have a look at the following articles to learn more.
