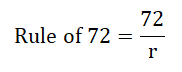Updated November 21, 2023
Rule of 72 Formula (Table of Contents)
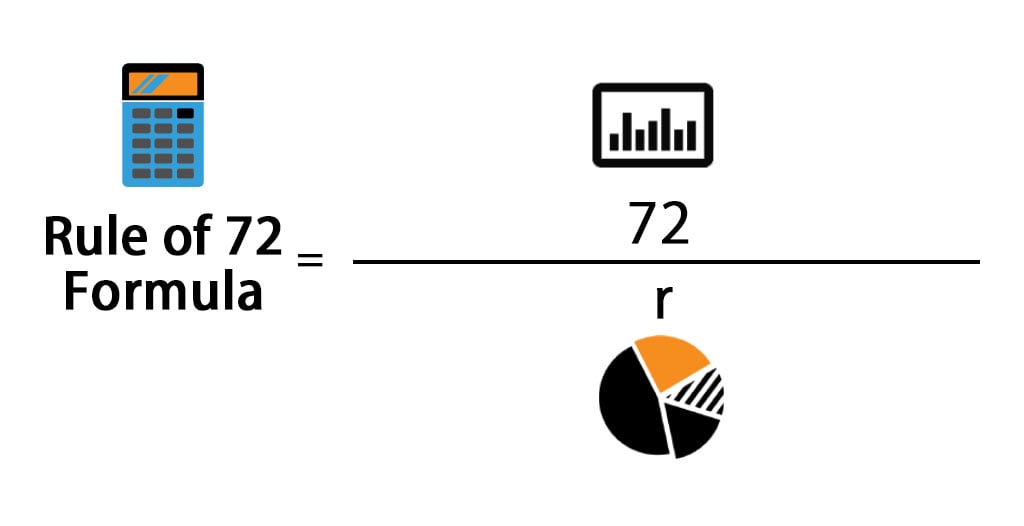
Rule of 72 Formula
Compound interest is the eighth wonder of the world. -Albert Einstein
The investment returns multiple times the invested amount if interest is calculated compounding.
For example – With 25% simple interest, in 3 years, it will provide 75% returns, but if we calculated it was compounding, it would give 95% returns.
Doing this calculation mentally will be strenuous, but Formula 72 can help in doing this calculation mentally.
Here’s the Rule of 72 formula –
Where,
r = the Rate of Return
The “Rule of 72 formula” is a shortcut method of calculating how long compounding interest will take to double an invested amount.
In other words –
It is a mathematical way to calculate the number of years it will take for investor money to double with compounding interest. In other words, it is easy to calculate how long investor money has to be invested to double at a specified interest rate.
Examples and Explanation
The Rule of 72 is used in finance or investment to quickly calculate the halving or doubling time through compound interest or inflation, respectively.
72 / [periodic interest rate] = [number of years to double principal amount]
Example #1
For example, using the Rule of 72, an investor who invests $2,000 at an interest rate of 8% per year will double their money in approximately 9 years.
By using the Formula of the 72 rule, we get –
- Rule of 72 = 72/r
- Rule of 72 = 72 / 8
- Rule of 72 = 9
Example #2
The investor, who invests $10,000 at a compounding interest rate of 4% per year, will double his money in approximately 18 years.
By using the Formula of the 72 rule, we get –
- Rule of 72 = 72/r
- Rule of 72 = 72 / 4
- Rule of 72 = 18
The same Formula can be used to find out the inflation effect on the amount –
72 / [inflation rate] = [number of years to half the principal amount]
Example #3
Using the same Rule of 72 formula stated above, an investor who invests $10,000 with an annual inflation rate of 1 % will lose half of their principal in 72 years.
By using the Formula of the 72 rule, we get –
- Rule of 72 = 72/r
- Rule of 72 = 72 / 1
- Rule of 72 = 72
The Rule of 72 can also be used to demonstrate the long-term effects of period fees on an investment, such as life insurance, mutual funds, and private equity funds. For example,
Example #4
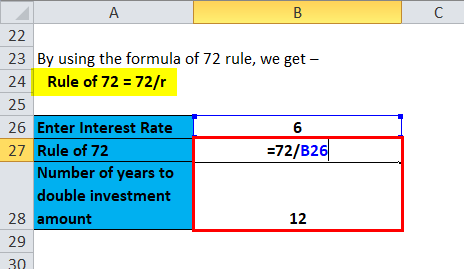
Not counting any appreciation of the underlying investments in the fund, a mutual fund with a 6% annual expense and loading fee on the principal invested will cut the principal in half over 12 years.
By using the Formula of the 72 rule, we get –
- Rule of 72 = 72/r
- Rule of 72 = 72/ 6
- Rule of 72 = 12
The Rule of 72 is an approximation. It is not exact. Indeed, the Rule of 72 is accompanied by the Rule of 70 and the Rule of 69, which are used similarly but are more accurate for smaller periodic interest rates. The Rule of 72 is popular because it is divisible by more numbers (i.e., possible interest rates).
| Interest Rate | Number of years invested to double the principal |
| 1 | 72 |
| 2 | 36 |
| 3 | 24 |
| 4 | 18 |
| 5 | 14 |
| 6 | 12 |
| 7 | 10 |
| 8 | 9 |
| 9 | 8 |
| 10 | 7 |
| 11 | 7 |
| 12 | 6 |
| 13 | 6 |
| 14 | 5 |
| 15 | 5 |
| 16 | 5 |
| 17 | 4 |
| 18 | 4 |
| 19 | 4 |
| 20 | 4 |
| 21 | 3 |
| 22 | 3 |
| 23 | 3 |
| 24 | 3 |
| 25 | 3 |
It is important to note that the Rule of 72 formula definition requires that the interest is compounded only annually. This method will not work for investments with a quarterly or semi-annual compounded interest rate as it is. If you want to use this method for investment returns for a quarterly or semi-annual compounded interest rate as it is, you will need to modify it.
Significance and Use
While this calculation is relatively straightforward with a calculator or spreadsheet, the Rule of 72 formula, which was derived before the 14th century, is also helpful for the mental calculation of the effects of compound interest.
Investors generally use this calculation method when calculating the differences among similar investments or the effect of inflation on an amount. Investors want to see their investments grow multiple times, so investors can think about investing in more opportunities in the future. This Rule can be used on any investment, not necessarily stock market or mutual fund investment.
Even the average American or any other citizen can use the Rule 72 method to calculate the amount of savings or money a retiree will have in a retirement fund or how much their share in a mutual fund or any other investment will be worth in five years, ten years or fifteen years. Rule 72 will help to calculate how long it will take to double your money in an investment corpus. In other words, it is an easy, very limited future value calculator that will calculate the value of an investor’s money in the future.
Rule 72 is a beneficial shortcut method because the investment equation for compounding interest is complicated and long. Anyone can use this simple Rule of 72 formula as a basic estimate for investment return calculations.
Rule of 72 Calculator
You can use the following Rule of 72 Calculator
| Rate of Return (r) | |
| Rule of 72 = | |
| Rule of 72 = |
|
|
Rule of 72 Formula in Excel (With Excel Template)
Here we will do the same example of the Rule of 72 in Excel. It is very easy and simple. You need to provide only one input, i.e., Rate of Return.
You can easily calculate the Rule of 72 using the Formula in the template provided.
By using the Formula of the 72 rule, we get –
Recommended Articles
This has been a guide to a Rule of 72 formula. Here we discuss its uses along with practical examples. We also provide the Rule of 72 Calculator with a downloadable Excel template. You may also look at the following articles to learn more –